1
$\begingroup$

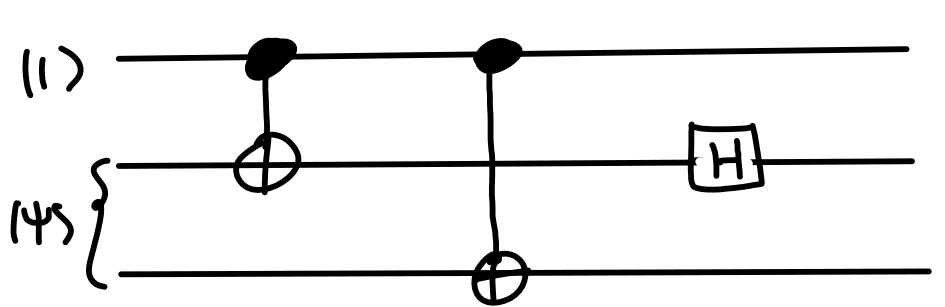
I am a bit confused by the following scenario:

Suppose we are given an entangled state $| \psi \rangle$ that has $2$ qubits (we can generalize to $n$ qubits).

enter image description here

Suppose we had a circuit like above. Because $| \psi \rangle$ is entangled, we cannot really CNOT each qubit, or Hadamard a single qubit. So how would we predict an outcome of such a circuit in general, mathematically?

And how could we extend this to more complex gates, like a CSWAP?

Improve this question
$\endgroup$
1
  • $\begingroup$ why do you think that $|\psi\rangle$ being entangled means you cannot apply the CNOT or Hadamard on each qubit? $\endgroup$
    – glS ♦
    Mar 31 at 11:53

1 Answer 1

Reset to default
1
$\begingroup$

Suppose $|\psi\rangle = (|00\rangle+|11\rangle)/\sqrt{2}$. The effect of the first CNOT is to flip the first qubit, therefore the entagled state becomes $|\psi'\rangle = (|10\rangle+|01\rangle)/\sqrt{2}$. Mathematically, you consider the whole state $|1\rangle|\psi\rangle = ..$ and apply the CNOT matrix to the first and second qubit (starting from the top).

Improve this answer
$\endgroup$
2
  • $\begingroup$ What if $| \psi \rangle = \frac{1}{\sqrt{3}}( | 00 \rangle + | 01 \rangle + | 11 \rangle )$ being applied in a CNOT? In this case, there are two kets that have a “0” qubit in them. $\endgroup$
    – Andrew Baker
    Mar 31 at 6:16
  • $\begingroup$ In that case you get $|\psi'\rangle = \frac{1}{\sqrt{3}}(|10\rangle + |11\rangle + |01\rangle)$. It is linear algebra, so you just apply the gate to each of the superposed elements of your state. $\endgroup$
    – Michele Amoretti
    Mar 31 at 7:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Not the answer you're looking for? Browse other questions tagged or ask your own question.