0
$\begingroup$

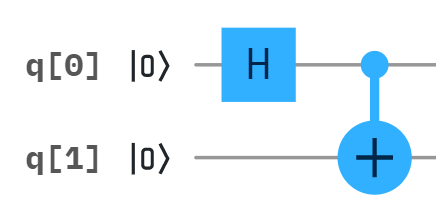
enter image description here

This is a picture from Wiki(https://en.wikipedia.org/wiki/Quantum_logic_gate). Can someone give me a simple example by using two qubits?

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ If you apply a unitary $F$ to the first qubit (q[0]), then your state would be the same as if you applied the conjugate transpose $F^\dagger$ to the second qubit (q[1]). Can you be more specific? $\endgroup$
    – Mark Spinelli
    Commented Apr 12, 2020 at 2:05
  • $\begingroup$ could you please edit the title to something that reflects what is being asked? $\endgroup$
    – glS ♦
    Commented Apr 13, 2020 at 8:01

1 Answer 1

Reset to default
2
$\begingroup$

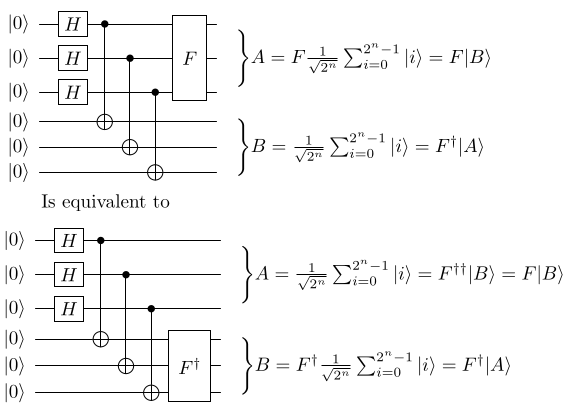
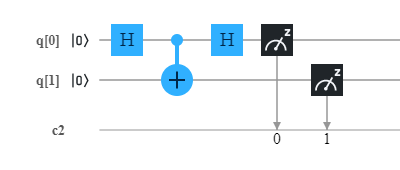
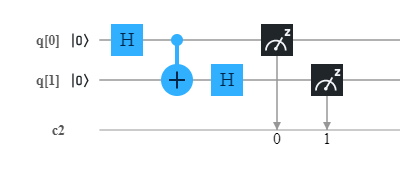
Since Fourier transform and inverse Fourier transform for one qubit is only Hadamard gate, for two qubit case following two circuits are equivalent.

First circuit (Fourier transform applied on qubit $q_0$)

Circuit 1

First circuit (inverse Fourier transform applied on qubit $q_1$)

Circuit 2

Both circuits return state

$$ |\psi\rangle = \frac{1}{2}(|00\rangle + |01\rangle + |10\rangle - |11\rangle). $$

EDIT: I have just realized that the gate $F$ is general unitary transformation and not the QFT (I was missleaded by F = Fourier). However, my example is also valid. It is a particular case for two qubits asked for in the question.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.