How are measurements on $Z$ and $X$ axes interpreted in the Bloch sphere?

Question

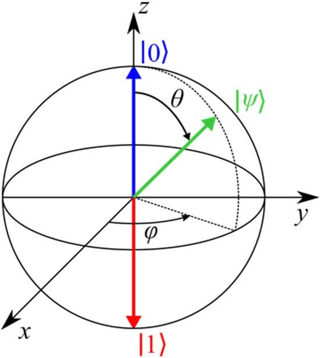
I'm having trouble understanding how the measurement on $z$ and $x$ axes can be interpreted in terms of the Bloch sphere representation.

.

I know that the state can be written as $$∣𝜓⟩=\cos(𝜃/2)|0⟩+\exp(i𝜙)\sin(𝜃/2)|1⟩,$$ and that to make a measurement we use the squared modulus of the inner product of the basis vector and the state vector. For example, we have $|⟨0|𝜓⟩|^2$ for a $z$ measurement.

Thinking in terms of the Bloch sphere, it is clear that a rotation by an angle $𝜙$ would not affect a $z$ measurement, and this is easy to prove mathematically. But this should also be the case for a $𝜃$ rotation on a $x$ measurement, right?

But when I try to prove it mathematically, I get

$$|⟨+|𝜓⟩|^2 = \frac{1}{\sqrt2}|(\cos(𝜃/2)+\exp(i𝜙)\sin(𝜃/2)|^2 \\= \frac{1}{\sqrt2}|(\cos(𝜃/2)+\cos(𝜙)\sin(𝜃/2)+i \sin(𝜙)\sin(𝜃/2)|^2,$$

which then can be written in the form of $a^2 + b^2$ where $a=\cos(𝜃/2)+\cos(𝜙)\sin(𝜃/2)$ and $b = \sin(𝜙)\sin(𝜃/2)$.

This means that $𝜃$ affects the probability of a $x$ measurement. I know I must be doing something wrong but what is it?

I also don't understand why x gate is a rotation around the x axis and not the y axis ?

Thanks in advance for your answer.

Answer 1

The $\theta$ and the $\phi$ angles are not equivalent in the Bloch sphere. First, they have different ranges -- one is $\pi$ and the other is $2\pi$. More importantly, $\phi$ is a rotation around a fixed axis, $z$, while $\theta$ is a rotation around a non-fixed axis that is moving with $\phi$. For $\phi=0$ this axis is $y$, for $\phi=\pi/2$ it is $x$, and for every other $\phi$ it is everything in between in the $x-y$ plane.

If pictorially we associate the probability of outcome to depend on the angle of the state's vector with the axis of measurement (the $z$ axis is the 0,1 measurement for example), then rotations around the $z$ axis should not affect the probabilities of 0,1 outcomes, and that's why the $\phi$ angle does not change this measurement. We cannot say the same about rotations by $\theta$ since it depends on the axis of rotation. In special cases though, for example if you set $\phi=\pi/2$, then the axis of $\theta$ rotation is $x$, then you should expect the +,- measurements along the $x$ axis not to depend on the $\theta$ (set $\phi=\pi/2$ and with basic trigo your equations should be telling you that).