Highest theoretical threshold to fight single-qubit depolarizing noise for noiseless error-correction

Question

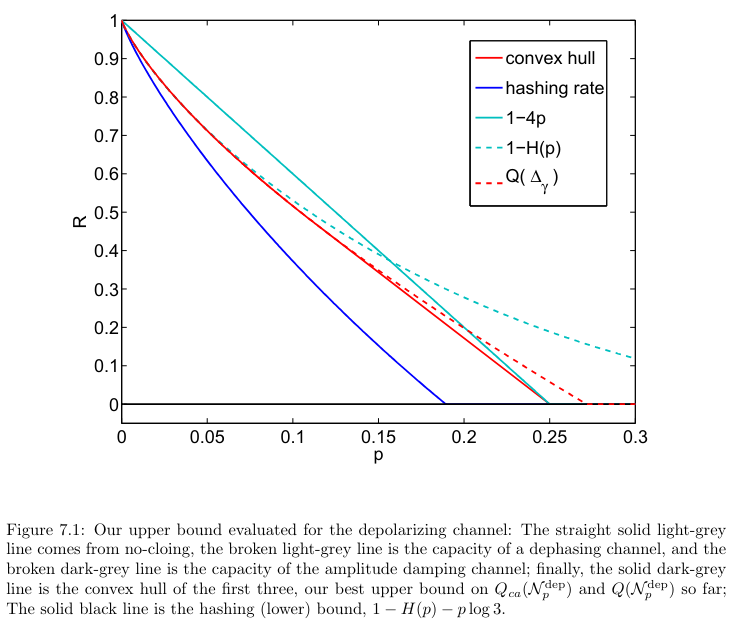
Let's consider that each qubit in the lab faces a single-qubit depolarizing channel $\mathcal{N}(\rho)=(1-p) \rho + p \mathbb{I}/2$.

Is there a theoretical result indicating the largest value of $p$ such that quantum error-correction can completely suppress the effect of the noise asymptotically (by encoding the qubit in logical qubits composed of sufficiently many physical qubits).

In different terms: is the maximum theoretical threshold to fight depolarizing noise known?

I am assuming that the implementation of error-correction is noiseless here.

Answer 1

One way of defining the quantum capacity of a quantum channel $\mathcal{E}$ is to ask, asymptotically, as $n\to \infty$, how much quantum information can I send with error rate going to $0$ over $\mathcal{E}^{\otimes n}$?

For the family of depolarizing channels $$\mathcal{E}_p (\rho) = (1-p) \rho + \frac{p}{3} X\rho X + \frac{p}{3}Y \rho Y + \frac{p}{3}Z \rho Z$$

Your question is equivalent to asking for the largest $p$ such that the quantum capacity is positive. Call this $p^*$. Unfortunately, the quantum capacity of the depolarizing channel is not known in closed form since the coherent information can be superadditive over multiple uses of the channel.** Lower bounds on $p^*$ by constructing coding schemes with a lower bound on their threshold.

Figure 7.1 in Graeme Smith's thesis plots some bounds on the capacity of the depolarizing channel

What we do know is the following:

• $p^* < 1/4$ by a no-cloning argument
• $p^* \ge .18929$ by a random coding argument (the "hashing bound")
• $p^* \ge 0.19086$ by using a concatenated code that utilizes degeneracy (Smith-Smolin 2007)

** For the most extreme example, there are channels that individually have zero capacity but together have finite capacity

Answer 2

If you assume the error correction to be noiseless and only consider a depolarizing channel on all qubits (say $p$ is the same on all qubits), then there will always be a probability of stabilizing a logical operator, this will be $\mathcal{O}(p^d)$, where $d$ is the code distance.

So if you are looking for the correction of an arbitrary number of errors, this will not be possible for any finite $p>0$.

Answer 3

Realistically, probably the zero-rate Hashing bound, so like 18-19%. Technically this could be violated? But I doubt by much.