One way of defining the quantum capacity of a quantum channel $\mathcal{E}$ is to ask, asymptotically, as $n\to \infty$, how much quantum information can I send with error rate going to $0$ over $\mathcal{E}^{\otimes n}$?
For the family of depolarizing channels $$\mathcal{E}_p (\rho) = (1-p) \rho + \frac{p}{3} X\rho X + \frac{p}{3}Y \rho Y + \frac{p}{3}Z \rho Z$$
Your question is equivalent to asking for the largest $p$ such that the quantum capacity is positive. Call this $p^*$. Unfortunately, the quantum capacity of the depolarizing channel is not known in closed form since the coherent information can be superadditive over multiple uses of the channel.** Lower bounds on $p^*$ by constructing coding schemes with a lower bound on their threshold.
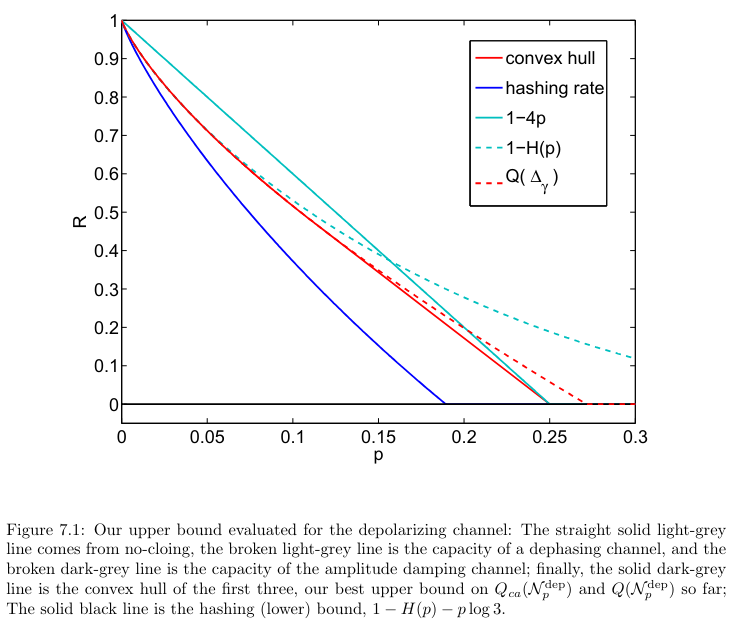
Figure 7.1 in Graeme Smith's thesis plots some bounds on the capacity of the depolarizing channel
What we do know is the following:
- $p^* < 1/4$ by a no-cloning argument
- $p^* \ge .18929$ by a random coding argument (the "hashing bound")
- $p^* \ge 0.19086$ by using a concatenated code that utilizes degeneracy (Smith-Smolin 2007)