How to create a quantum circuit transforming $α|00⟩ + β|11⟩$ to $β|00⟩-α|01⟩+β|10⟩+α|11⟩$

Question

Starting from an unknown state $α|00⟩ + β|11⟩$, where $\alpha,\beta$ are properly normalized, how can I create a circuit that transforms that state to a $\frac{1}{\sqrt{2}} (β|00⟩-α|01⟩+β|10⟩+α|11⟩)$ state?

Answer 1

Note that the output state can be written as,

$\frac{1}{\sqrt{2}}(\beta|00\rangle - \alpha|01\rangle + \beta|10\rangle + \alpha|11\rangle)$

= $\frac{1}{\sqrt{2}}(\beta(|00\rangle + |10\rangle) - \alpha(|01\rangle - |11\rangle))$

= $\frac{1}{\sqrt{2}}(\beta(|0\rangle + |1\rangle)|0\rangle - \alpha(|0\rangle - |1\rangle)|1\rangle)$

= $\beta|+\rangle|0\rangle - \alpha|-\rangle|1\rangle$

= $\beta(H|0\rangle)|0\rangle - \alpha(H|1\rangle)|1\rangle$

Where $|+\rangle$ and $|-\rangle$ are the two orthogonal x-basis states: $$|+\rangle = \frac{1}{\sqrt{2}}(|0\rangle + |1\rangle) = H|0\rangle$$ $$|-\rangle = \frac{1}{\sqrt{2}}(|0\rangle - |1\rangle) = H|1\rangle$$​ And $H$ is the Hadamard gate.

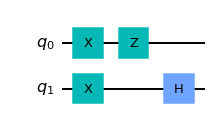
To create the circuit first note that in the final state $\alpha$ is amplitude of the state $|11\rangle$ instead of $|00\rangle$. The opposite happens with $\beta$. It is a bit-flip operation. So, we need to apply $X$-gate to both qubits. The resulting state will be $$\beta|00\rangle + \alpha|11\rangle$$ Then we need to change the sign of the $|11\rangle$ state. It is a phase-flip operation. So, we need to apply $Z$-gate to any one of the qubits. Now we have, $$\beta|00\rangle - \alpha|11\rangle$$ Finally, we need to apply $H$-gate to the second qubit.

Answer 2

Since the circuit U is linear (as always) you can investigate its action to each input independently:

$$ \begin{align} U\bigl(\alpha|00\rangle + \beta|11\rangle\bigr) &= \alpha U|00\rangle + \beta U|11\rangle\\ &=\alpha\frac1{\sqrt{2}}\bigl(-|01\rangle +|11\rangle\bigr) + \beta\frac1{\sqrt{2}}\bigl(|00\rangle + |10\rangle\bigr) \end{align} $$

You, therefore, need a circuit that acts as:

$$ \begin{align} U|00\rangle &= \frac1{\sqrt{2}}\bigl(-|01\rangle + |11\rangle\bigr) \\ U|11\rangle &= \frac1{\sqrt{2}}\bigl(|00\rangle + |10\rangle\bigr) \end{align} $$

It is obvious that there is no one unique circuit that achieves this since you don't know its action on $|01\rangle$ and $|10\rangle$.

Next you can check if the right hand side contains entangled qubits or not so that you know if you need entangling (two-qubit) gates or not. Obviously, they are not entangled:

$$ \begin{align} -|01\rangle +|11\rangle &= -\bigr(|0\rangle -|1\rangle\bigl)|1\rangle = -\bigr(H|1\rangle\bigl)|1\rangle\\ |00\rangle +|10\rangle &= \bigr(|0\rangle +|1\rangle\bigl)|0\rangle = \bigr(H|0\rangle\bigl)|0\rangle\\ \end{align} $$

Therefore, the action of $U$ is:

$$ \begin{align} U|00\rangle &= \frac1{\sqrt{2}}\bigl(-|01\rangle + |11\rangle\bigr) \\ &= -HI|11\rangle \\ &= HZ|11\rangle \\ &= (HZ)(XX)|00\rangle \end{align} $$

and

$$ \begin{align} U|11\rangle &= \frac1{\sqrt{2}}\bigl(|00\rangle + |10\rangle\bigr) \\ &= HI|00\rangle\\ &= (HI)(XX)|11\rangle \\ &= (HZ)(XX)|11\rangle \end{align} $$

where in the last step we replaced $I$ with $Z$ since it has no effect on the state $|00\rangle$.

So one possible circuit that transforms your input state to the desired output state is a circuit with two $X$ gates followed by a $H$ gate on one qubit and a $Z$ gate on the other.