On page 35 in Nielsen and Chuang, it's said that for the following quantum circuit implementing the general Deutsch–Jozsa algorithm:
Next, the function $f$ is evaluated (by Bob) using $U_f$, giving $$\left|\psi_2\right\rangle=\sum_x\frac{(-1)^{f(x)}|x\rangle}{\sqrt{2}^n}\left[\frac{|0\rangle-|1\rangle}{\sqrt{2}}\right]$$
I'm confused about where the $(−1)^{f(x)}$ come from.
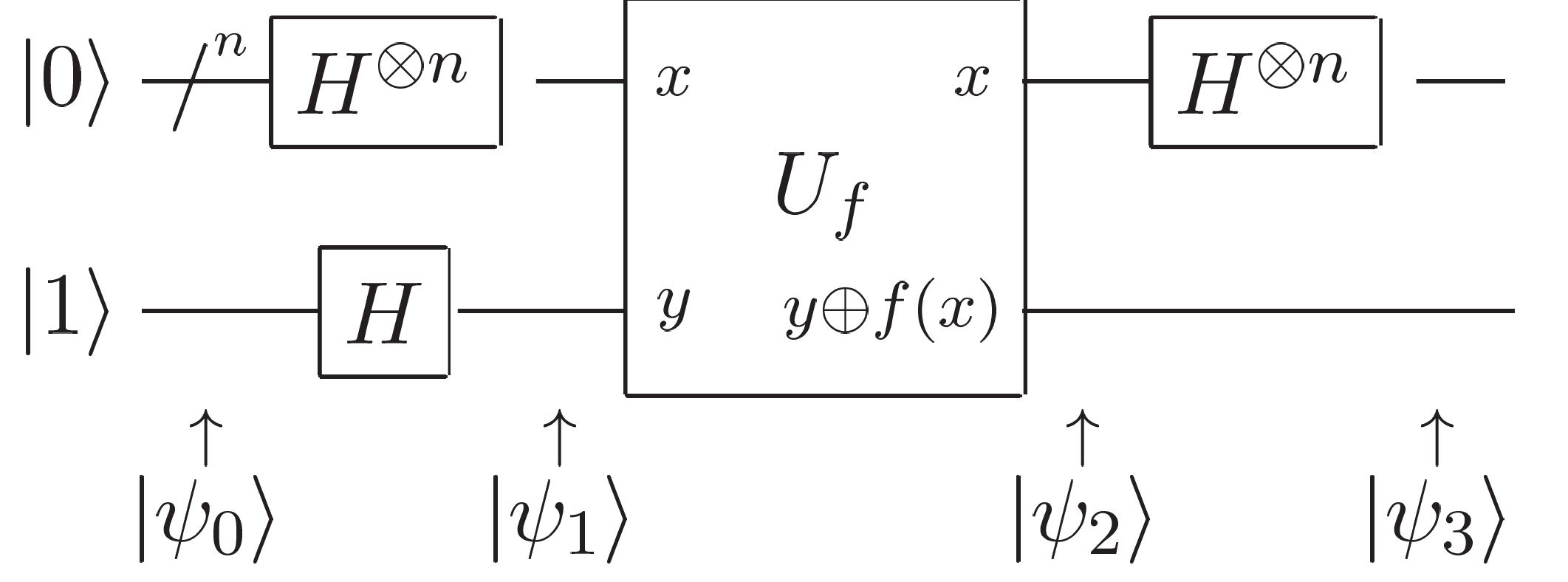
First of all, if we write down $\left|\psi_1\right\rangle$, we get: $$\left|\psi_1\right\rangle=\frac{1}{\sqrt{2}^n}\sum_x|x\rangle\left[\frac{|0\rangle-|1\rangle}{\sqrt{2}}\right].$$ Applying $f$ on this state gives us: $$\left|\psi_2\right\rangle=\frac{1}{\sqrt{2}^n}\sum_x|x\rangle\left[\frac{|f(x)\rangle-|1\oplus f(x)\rangle}{\sqrt{2}}\right].$$ Note that $f(x)$ is a bit. As such, $f(x)\oplus 1$ is actually $f(x)$ on which one applied a NOT gate. So, for a given $x$, if $f(x)=0$, then we can write: $$|f(x)\rangle-|1\oplus f(x)\rangle = |0\rangle-|1\rangle$$ while we can write, if $f(x)=1$: $$|f(x)\rangle-|1\oplus f(x)\rangle = |1\rangle-|0\rangle = -\left(|0\rangle-|1\rangle\right).$$ Thus, it is completely equivalent to write, in the general case: $$|f(x)\rangle-|1\oplus f(x)\rangle = (-1)^{f(x)}\left(|0\rangle-|1\rangle\right).$$ Indeed, in the case $f(x)=0$, the $(-1)$ will disappear, while in the case $f(x)=1$, it will stay, just like in the two previous equations. This means that we can rewrite the state as: $$\left|\psi_2\right\rangle=\frac{1}{\sqrt{2}^n}\sum_x|x\rangle(-1)^{f(x)}\left[\frac{|0\rangle-|1\rangle}{\sqrt{2}}\right]$$ which is the state described in Nielsen and Chuang.
