Suppose I want to perform the time-evolution simulation on the following Hamiltonians:
$$ H_{1} = X_1+ Y_2 + Z_1\otimes Z_2 \\ H_{2} = X_1\otimes Y_2 + Z_1\otimes Z_2 $$
Where $X,Y,Z$ are Pauli matrices. Since $[X_1,Y_2] = 0$, I can simultaneously perform the time evolution simulation of $X_1$ and $Y_2$. Thus, it seems like both Hamiltonians could be simulated using the following circuit ($\pi$ is just a random number):
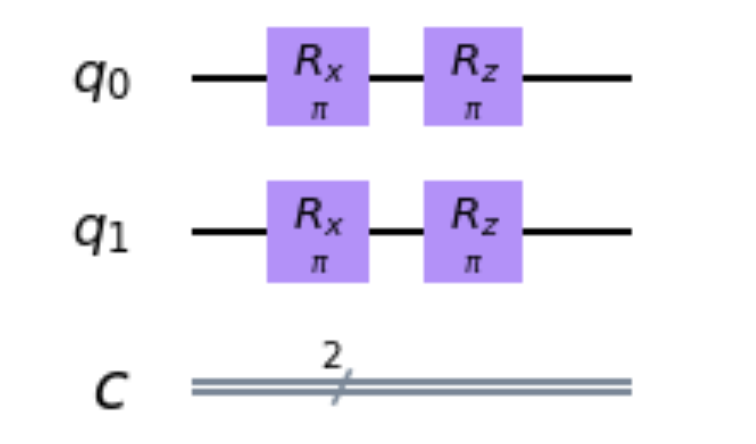
Is this circuit looks right? If so, how can I tell the difference between these two Hamiltonians just by looking at the circuit?
