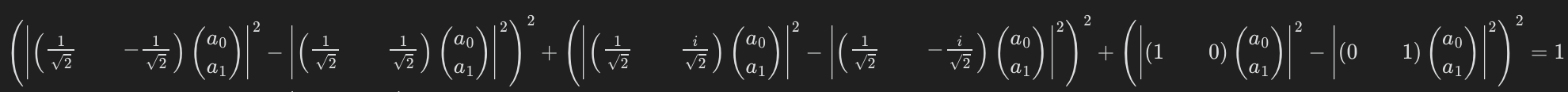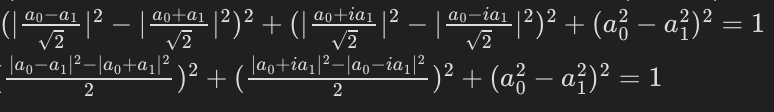I am trying to solve an exercise, but I can't seem to get it to work. I have to show $$\langle \sigma_x \rangle^2 + \langle \sigma_y \rangle^2+\langle \sigma_z \rangle^2 =1 $$
for $|\psi\rangle = \cos\theta|0\rangle + \sin\theta|1\rangle$. I first expand upon the rule, by actually computing the probabilities for each base.
I assume my mistake lies here.
When inserting $a_0 = \cos\theta$ and $a_1 = \sin\theta$ I get this:
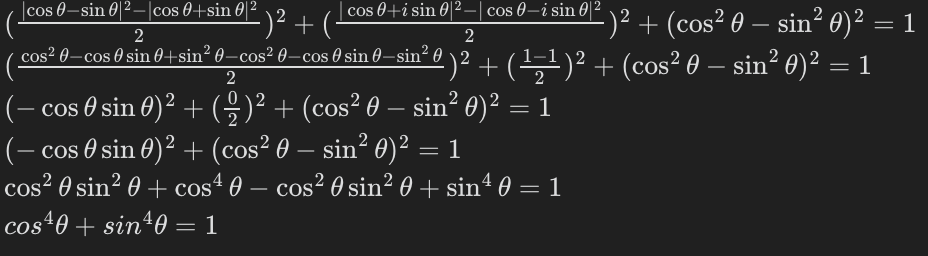
This is incorrect though, because the relationship at the beginning should, according to the exercise, hold for all $\theta$ and not just when $\theta = n\pi$ $\forall n \in \mathbb N$, because it asks me to verify it for that specific state ($|\psi\rangle= \cos\theta|0\rangle + \sin\theta|1\rangle$). Can anyone help me out here? Thanks, Tom