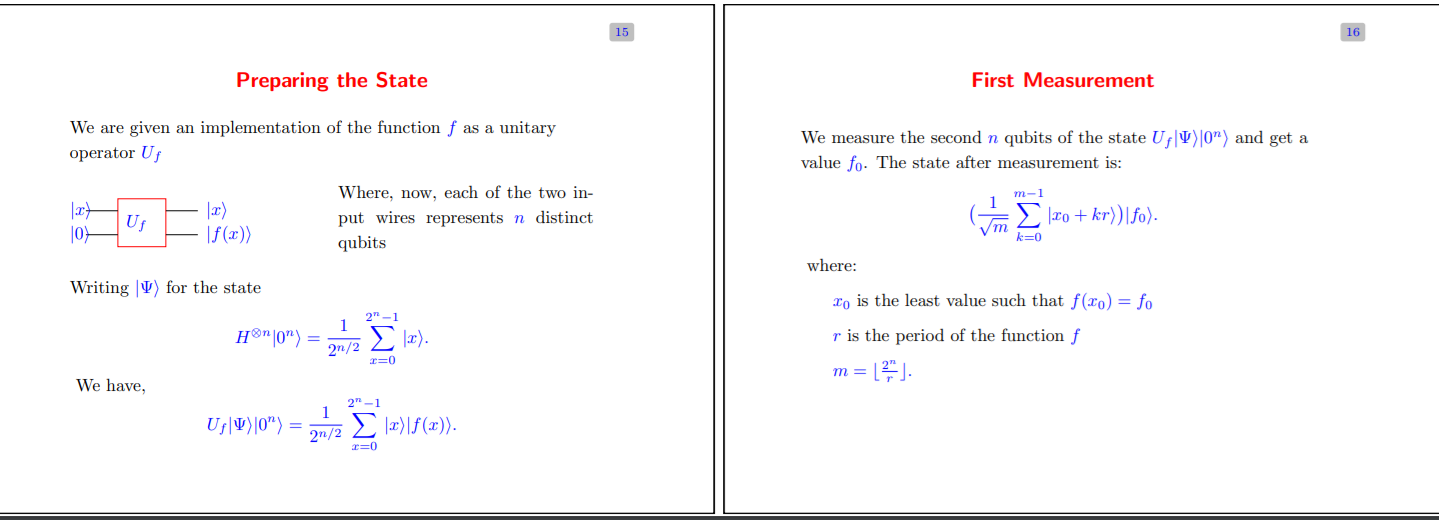
In the period finding algorithm (see the picture), through the standard procedure in quantum computing algorithms, we have $$U_f|\Psi\rangle|0^n\rangle=\frac{1}{\sqrt{2^n}}\left(\sum_{x=0}^{2^n-1}|x\rangle\right)\otimes|f(x)\rangle.$$ Then the next key step, we apply the measurement. My question is, if we apply the measurement with computational basis $P_m$ only in the second component (the space where $|f(x)\rangle$ lives), then this measurement with respect to the whole composite system is $I\otimes P_m$.
Then follow this viewpoint, the "first" component should not be changed after the measurement right? (Since $\left(\sum_{x=0}^{2^n-1}|x\rangle\right)$ and $|f(x)\rangle$ are clearly not entangled, i.e. it can be written as the form $|\phi\rangle\otimes|\psi\rangle$). Therefore the resulting state should be something like $\underbrace{\left(\sum_{x=0}^{2^n-1}|x\rangle\right)}_{\text{unchanged}}\otimes|f_0\rangle$.
Where did I have the mistake?