Is there a separable state that is furthest away from an entangled one?

Question

I see a lot of Venn kind of diagrams to depict the distinction between separable and entangled mixed states. Like this one (apologize my poor "paint"ings):

$\hskip2.0in$

So there is an entangled state $\rho$. It is entangled because it is not inside the set of separable states. My question is inspired by the picture:

Is there a separable state that is furthest away from the entangled one?

I tried to indicate it in red, but can we calculate the separable state?

To be concrete: What is the separable state furthest away from a GHZ state:$(|00\cdots 0\rangle+|11\cdots 1\rangle)/\sqrt2$...

Answer 1

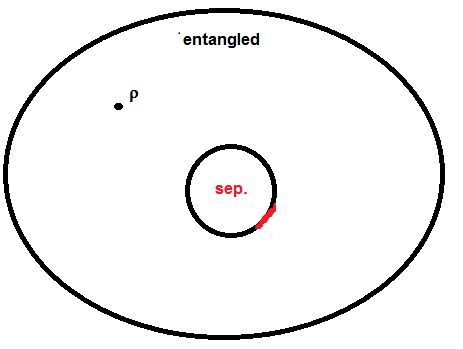
Here's an example based on entanglement witnesses. Let $$ |\psi\rangle=\frac{1}{\sqrt{2}}(|000\rangle+|111\rangle). $$ The aim is to define an operator $W$ such that $\text{Tr}(W\rho_\text{sep})\geq 0$ for all separable states $\rho_\text{sep}$. That means for any $\rho$ such that $\text{Tr}(W\rho)<0$, it must be entangled.

An easy way to define such an operator is $$ W=\alpha I-|\psi\rangle\langle\psi|, $$ where $$ \alpha=\max_{\rho_\text{sep}}\langle\psi|\rho_\text{sep}|\psi\rangle. $$ In this particular case, the maximisation is achieved with $\rho_\text{sep}=|0\rangle\langle 0|^{\otimes 3}$, I believe, so $\alpha=\frac12$.

Of course, $\text{Tr}(W|\psi\rangle\langle\psi|)=\alpha-1=-\frac12$, so we see that it's entangled.

Now consider any other state $\rho$. We can think of the value $\text{Tr}(W\rho)$ as measuring distance. The smallest possible value is $-\frac12$, and if you have that, you've got the state $|\psi\rangle$. The larger the value, there is a sense of getting further away from $|\psi\rangle$. Now, the maximum value of $\text{Tr}(W\rho)$ is $\alpha=\frac12$, which is achieved by $\langle\psi|\rho|\psi\rangle=0$. So this categorises the states that are furthest away according to this specific measure. There are separable states which are orthogonal to $|\psi\rangle$, such as $|001\rangle$, and so they can be considered the furthest separable states.

Answer 2

Whenever under the distance measure you are using, the set of separable states is closed, there is a furthest point - this is a feature of closed sets. This is certainly the case whenever the distance measure is continuous relative to any other measure relative to which the separable states are closed, such as the trace norm distance.

In particular, there is always a furthest state as measured by the trace norm distance.

Answer 3

A somewhat informal comment.

Some intuition can be developed from the fact that the pure states are rays in the complex projective space (subject to an additional normalization constraint). The most 'different' the two vectors in such a space can be is 'perpendicular' ⁠— if you keep $v_1$ moving away from $v_2$ in a certain plane, the distance will start increasing once you pass the perpendicular direction (think about the distance along the arc between two points on a circle, or about the maximum angle between any two lines passing through the origin). We also know that, because of the normalization condition, there is a maximum value for the scalar product between two vectors ⁠— given by the vectors' norm. Thus, in the sense of a particular norm, the two states can be as close as $0$, and as far as $1$ apart from each other.

Note that the trace norm distance between the states, which I was implicitly referring to, is precisely the one inherited from the scalar product of two vectors in the complex projective space (which, in turn, is inherited from the usual scalar product of vectors in $\mathbb{C}^n$): while for vectors we have $d(|\psi\rangle,|\psi'\rangle)=|\langle \psi | \psi' \rangle|$, for the corresponding pure density matrices we have $d(|\psi\rangle\langle\psi|, |\psi'\rangle\langle\psi'|) =\operatorname{tr} (|\psi\rangle\langle\psi||\psi'\rangle\langle\psi'| )$, which is obtained by squaring the previous expression.

Answer 4

$\newcommand{\bra}[1]{\left<#1\right|}\newcommand{\ket}[1]{\left|#1\right>}\newcommand{\bk}[2]{\left<#1\middle|#2\right>}\newcommand{\bke}[3]{\left<#1\middle|#2\middle|#3\right>}$

Let $\ket{GHZ}$ be a GHZ state with $n$ qubits. So thanks to Norbert's answer I found the following separable pure states that maximize the trace distance: $$ \ket\psi=\frac1{2^{n/2}}\otimes_{k=1}^n(\ket0 +\exp{\left(i\pi f(k)\right)} \ket1), $$ with $\sum_{k=1}^n f(k)=1$. By that we get the coefficient of $\ket{00\cdots 0}$ to be $1$ and the one of $\ket{11\cdots 1}$ to be $-1$. Therefore $\bk\psi{GHZ}=0$ holds. They all have trace distance: $$ \frac12\sum |\lambda_i|=1, $$ where $\lambda_i$ are the eigenvalues of $\left(\ket{GHZ}\bra{GHZ}-\ket\psi\bra\psi\right)$. Both states share the same permutation symmetry if $f(k)=1/n$...