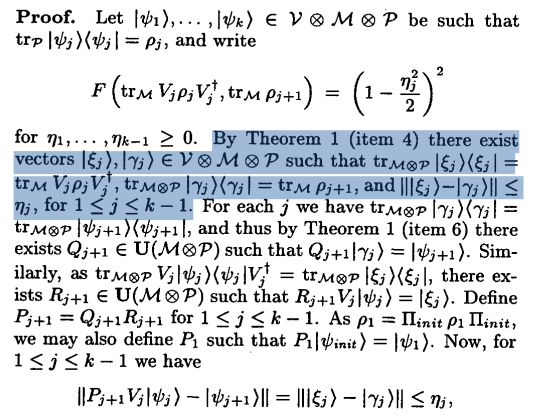Consider two density operators $\rho, \xi \in \mathrm{D}(\mathcal{H} \otimes \mathcal{L})$ where $\mathrm{dim}(\mathcal{H}) \le \mathrm{dim}(\mathcal{L})$. Define
\begin{align*} \epsilon := \mathrm{min}\{\|\,|\phi\rangle - |\psi\rangle\,\| : &|\phi\rangle,|\psi\rangle\,\in \mathcal{H} \otimes\mathcal{L}, \\&\mathrm{Tr}_{\mathcal{L}}(|\phi\rangle\langle\phi|)=\mathrm{Tr}_{\mathcal{L}}(\rho), \mathrm{Tr}_{\mathcal{L}}(|\psi\rangle\langle\psi|)=\mathrm{Tr}_{\mathcal{L}}(\xi) \}. \end{align*} Then it follows from Uhlmann's theorem that $\mathrm{F}(\mathrm{Tr}_{\mathcal{L}}(\rho), \mathrm{Tr}_{\mathcal{L}}(\xi)) = (1 - \frac{\epsilon^2}{2})^2 $.
Now, in this paper, the authors define $\eta \in \mathbb{R}_{\ge 0}$ by requiring $\mathrm{F}(\mathrm{Tr}_{\mathcal{L}}(\rho), \mathrm{Tr}_{\mathcal{L}}(\xi)) = (1 - \frac{\eta^2}{2})^2$. They proceed to claim, using the above result, that there must exist purifications $|\phi\rangle, |\psi\rangle \in \mathcal{H}\otimes\mathcal{L}$ of $\mathrm{Tr}_{\mathcal{L}}(\rho)$ and $\mathrm{Tr}_{\mathcal{L}}(\xi)$ respectively such that $\|\,|\phi\rangle - |\psi\rangle\,\| \le \eta$.
However, shouldn't the inequality be the other way around, since non-ideal purifications would result in $\|\,|\phi\rangle - |\psi\rangle\,\|$ being greater than the minimum, which in this case must be $\eta$? And why can't we go for the stronger equality, since we can always pick the $|\phi\rangle, |\psi\rangle$ that give $\mathrm{min}(\|\,|\phi\rangle - |\psi\rangle\,\|) = \eta$?
I am sure I am having some trivial misunderstanding of elementary algebra here, but I genuinely can't seem to figure out what.