How to know whether or not we have an X-cut or Z-cut without information on the boundaries

Question

I am reading this paper, in particular the part where X-cut and Z-cut are being defined. My question is highly connected (but different) to this one I previously asked.

In this paper, they show the following image with the following quote. I have but in bold the part that confuses me.

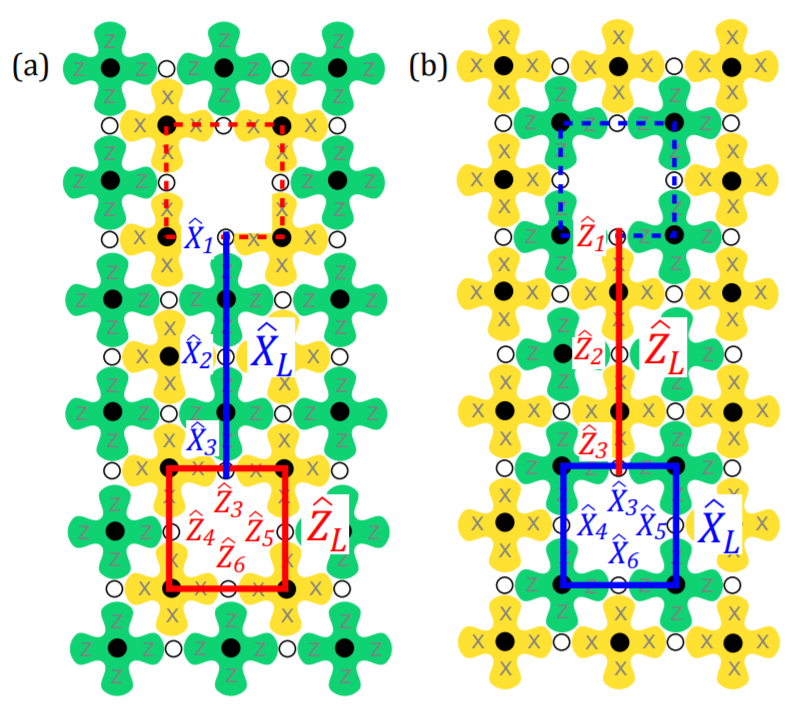
(Color online) (a) A double Z-cut and (b) a double X-cut qubit, formed in a large array by turning off two measure-Z and two measure-X qubits, respectively; the array is assumed to extend outwards indefinitely. For the double Z-cut qubit the logical operators comprise an XˆL = ˆX1 ˆX2 ˆX3 chain that links one Z-cut hole’s internal X boundary to the other, and a ZˆL = ˆZ3 ˆZ4 ˆZ5 ˆZ6 loop that encloses the lower Z- cut hole. For the X-cut qubit we have the ˆZL = ˆZ1 ˆZ2 ˆZ3 chain that links the two internal X-cut holes’ Z boundaries and the XˆL = ˆX3 ˆX4 ˆX5 ˆX6 loop that encloses the lower X-cut hole. The XˆL and ZˆL operator chains share one data qubit, data XˆL qubit 3 for both examples, so the operators anti-commute. Note that the loop operators ( ˆZL for the Z-cut qubit and for the X-cut qubit) can surround either of the two holes in the qubit, as discussed in the text.

What confuses me is that to know if we add degree of freedom to the surface, we need to have informations about boundary conditions. If it appeared that the boundary where all composed of $X$ generator, on figure a), the stabilizer removed would be a product of the others (hence no logical qubit would have been created).

In this quotation it says that the array extends in an infinite manner. What does that mean? For me if I imagine an infinite array I would see the two $X$ stabilizers removed here as the product of all the other $X$ stabilizers (hence no degree of freedom is created).

My questions are then:

1. How to understand the boundary condition they are using to create this logical qubit. What did they precisely mean in the text.
2. Is there a standard convention to assume when reading surface code paper about the boundary condition they are using (if they are not explicitly specifying it).

Answer 1

How to understand the boundary condition they are using to create this logical qubit. What did they precisely mean in the text.

They just mean that the boundaries are unspecified but far enough way to be irrelevant. It could be an infinite grid, it could be a really big donut, it could be a really big square patch with a variety of X or Z cuts running around its side... they're not going to make any assumptions that depend on this detail. All the relevant information is local to the holes.

Is there a standard convention to assume when reading surface code paper about the boundary condition they are using (if they are not explicitly specifying it).

If the boundary isn't specified, you should be careful not to do any analysis that depends on what it is. Some questions, like "how many logical qubits are here", can depend on the choice. Ideally you can rework those questions into things like "how many logical qubits whose observables don't leave this area are here".

Most modern surface code papers use lattice surgery instead of braiding, so this doesn't really come up anymore. Lattice surgery puts the qubits inside boundaries, instead of outside holes.