1
$\begingroup$

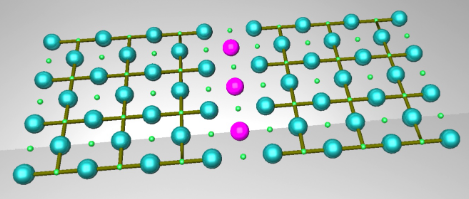
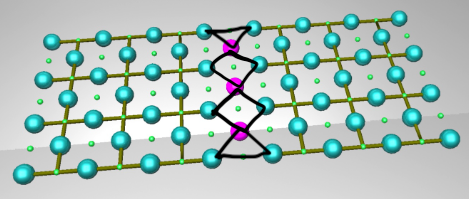
In the paper Surface code quantum computing by lattice surgery, for rough merging, they state that measuring the $X$ stabilisers at the boundary is equivalent to $X_{L}X_{L}$.

What I don't understand is what the new $X$ stabiliser measurements spanning the old boundary are meant to be. and how they're product gives these logical operators.

Do they mean that, using the 3 pink data qubits, they construct 3 new $X$ stabilizer measurements, and then the product of those 3 stabilizers would be equivalent to 2 logical $X$ operators running parallel to the old boundary? If this is the case, then why would the rounds of error correction we do on both the 3 qubits and 2 surfaces allow us to somehow arrive at this

If they are taking the existing $X$ stabilisers, then I don't see how the product of them even givens you the product of the 2 logical $X$ operators of each surface, given they act on different qubits, unless the idea is that the data qubits in the boundaries of the lattice disappear.

image showing lattice merging from paper

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

The $X$ stabilizers to be measured are the ones indicated. You have two three-qubit stabilizers at the top and bottom and two four-qubit stabilizers. enter image description here

Note that each pink qubit is acted on by two stabilizer measurements. Hence that the product of all these stabilizers is equal to two columns of $X$ measurements, one on the rightmost column of the left patch and one on the leftmost column of the right patch. This is the measurement $X_LX_L$.

Improve this answer
$\endgroup$
9
  • $\begingroup$ Thank you for your answer. Ok so it was my first guess. But how does the measurement actually give us $X_{L}X_{L}$? As in my question, I can clearly see that the product of the operators of the stabilisers will indeed give the product of those two logical operators. But the measurement of stabilisers doesn't cancel overlapping operators in the same way the product does. $\endgroup$
    – GaussStrife
    Commented Aug 14 at 7:38
  • $\begingroup$ ie if I measure those 4 stabilisers, it will collapse the two surfaces into an eigenstate of the 4 of them. But that wouldn't be the same as just measuring the logical operators, and the eigenstate in question wouldn't even be a +1 eigenstate, correct? $\endgroup$
    – GaussStrife
    Commented Aug 14 at 7:40
  • 1
    $\begingroup$ You get the four individual outcomes of $\pm 1$ for each stabilizer (these are indeed random) but the product of those four outcomes is the same as the outcome of the observable $X_LX_L$ which may or may not be random depending on the joint state of the two patches. $\endgroup$
    – Navneeth Ramakrishnan
    Commented Aug 19 at 10:22
  • 1
    $\begingroup$ As for your first comment, consider measuring two Pauli observables $P_1, P_2$ and obtaining $+1$ for both or $-1$ for both (i.e. the product is $+1$). This means that you apply the projector $\frac{1+P_1}{2}\frac{1+P_2}{2} + \frac{1-P_1}{2}\frac{1-P_2}{2} = \frac{1 + P_1P_2}{2}$ on the state. Replace $P_i$ with the stabilizers here to see why you can ignore the $X$ operations on the pink qubits. $\endgroup$
    – Navneeth Ramakrishnan
    Commented Aug 19 at 11:43
  • 1
    $\begingroup$ Yes, you're correct. $\endgroup$
    – Navneeth Ramakrishnan
    Commented Aug 30 at 4:34

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.