How does the propogation of bi-product operators through the CNOT gate in Gate Teleportation give this circuit?

Question

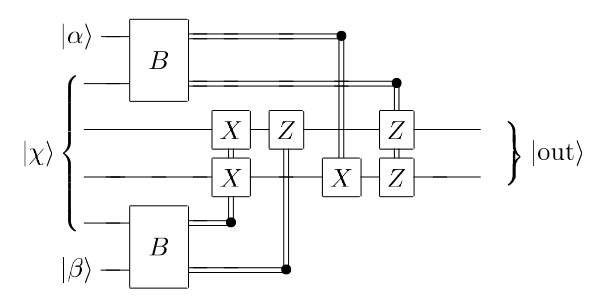
I am looking at Quantum Teleportation is a Universal Computational Primitive, and I can't follow how they derive the circuit for Fig 2.

I understand that the CNOT gate between the second qubit of the first bell pair and the first of the second occurrs after the bi-product operators acting on qubits 3 and 4. We can then use commutation to move it earlier in the circuit. However, taking a CNOT gate and moving it through $X$ and $Z$ bi-product operations, we get (going from left to right, but it doesn't matter)

$$C_{3,4}Z_{3}X_{3}\to Z_{3}X_{3}X_{4}C_{3,4} $$ given classical control by $|\alpha\rangle $ and $$C_{3,4}Z_{4}X_{4}\to Z_{3}Z_{4}X_{4}C_{3,4} $$ given classical control by $|\beta\rangle$

Even taking the control and target for the EPR pairs as flipped, we get

$$C_{4,3}Z_{3}X_{3}\to Z_{3}Z_{4}X_{3}C_{3,4} $$ given classical control by $|\alpha\rangle $ and $$C_{4,3}Z_{4}X_{4}\to X_{3}X_{4}Z_{4}C_{3,4} $$ given classical control by $|\beta\rangle$

But they have

$$C_{4,3}Z_{3}X_{3}\to Z_{3}Z_{4}X_{4}C_{3,4} $$ given classical control by $|\alpha\rangle $ and $$C_{3,4}Z_{4}X_{4}\to X_{3}X_{4}Z_{3}C_{3,4} $$ given classical control by $|\beta\rangle$

Now given these are all LOCC gates, I know that in certain instances, depending on the measurement outcomes, these would cancel to give the usual single $X$ and $Z$ operations. Additionally, they are bi-product operations, so it doesn't really matter what happens with them. But I would like to understand why I am getting something different than they are for the new LOCC operations with classical control.

Answer 1

Are you doing CX 2 3 or CX 3 2 when preparing the middle state? The corrections are for CX 3 2.

import stim


circuit = stim.Circuit("""
    RX 1 3
    R 2 4
    TICK

    CX 1 2 3 4
    TICK

    CX 3 2
    TICK
    
    CX 1 0 5 4
    TICK

    M 0 4
    MX 1 5
    TICK
    
    CX rec[-3] 2 rec[-3] 3
    CZ rec[-1] 3
    CX rec[-4] 2
    CZ rec[-2] 2 rec[-2] 3
""")

assert circuit.has_all_flows([
    stim.Flow("X0 -> X2"),
    stim.Flow("Z0 -> Z2*Z3"),
    stim.Flow("X5 -> X2*X3"),
    stim.Flow("Z5 -> Z3"),
])
print(circuit.diagram())

                        /------------------------\
q0: --------X-M:rec[0]-----------------------------
            |
q1: -RX-@---@-MX:rec[2]----------------------------
        |
q2: -R--X-X-------------X^rec[1]-X^rec[0]-Z^rec[2]-
          |
q3: -RX-@-@-------------X^rec[1]-Z^rec[3]-Z^rec[2]-
        |
q4: -R--X---X-M:rec[1]-----------------------------
            |
q5: --------@-MX:rec[3]----------------------------
                        \------------------------/