Does measuring the operator $XX$ preserve superpositions in a given two-qubit state?

Question

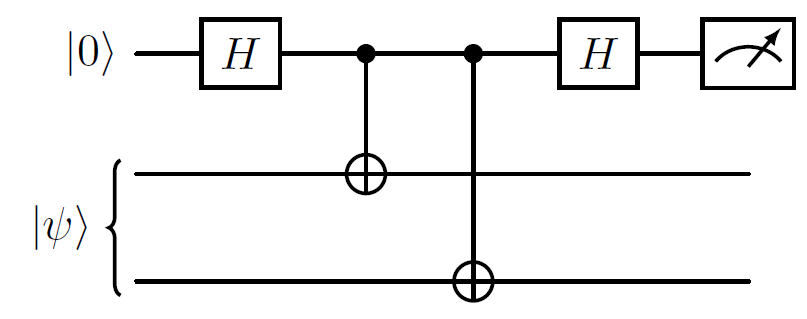
Suppose I have a state $$\vert\psi\rangle = a\left(\frac{1}{\sqrt{2}}\vert\Phi^+\rangle + \frac{1}{\sqrt{2}}\vert\Phi^-\rangle\right) + b\left(\frac{1}{\sqrt{2}}\vert\Psi^+\rangle + \frac{1}{\sqrt{2}}\vert\Psi^-\rangle\right),$$

where I have used the Bell basis states. Suppose I measure the operator $XX$ and obtain $+1$. Is my post measurement state now

$$a\vert\Phi^+\rangle + b\vert\Psi^+\rangle$$

i.e. does such a measurement preserve coherence? Similarly for the $-1$ outcome, is the state

$$a\vert\Phi^-\rangle + b\vert\Psi^-\rangle$$

From the answer, it seems like it depends on how the measurement is implemented. How should one implement the measurement to obtain this result (preserving the coherence)?

Answer 1

TLDR: yes.

That might depend on exactly how you implement the measurement, but if you do it properly, using a non-destructive measurement, then yes. Projective measurements project onto their eigenspaces. If that space is not rank 1, then the superposition within the subspace is preserved.

The circuit that you would use is:

This is just the standard circuit for measuring an observable $\sigma=\sigma_1\otimes\sigma_2\otimes\ldots$ where $\sigma^2=I$.