Definition of a NISQ device with respect to qubit counts and error rates

Question

How do we define whether a device is a noisy intermediate-scale quantum (NISQ) device with respect to number of qubits and their error rates? Does it make sense to do this? I believe I once saw a definition of a NISQ device as one with on the order of $n \le 10^5$ qubits and single qubit error rates on the order of $\epsilon \ge 10^{-4}$ – are there any references that attempt to define the 'intermediate-scale' part of NISQ? As far as I'm aware, John Preskill did not provide any explicit definitions (this seems to have been prudent at the time, perhaps it still is).

Attempting to unpack things a bit, it seems that a NISQ device must be one that is designed to leverage noisy, not logical (error-corrected), qubits. If we are to believe that, then the obvious question is how many logical qubits do we need to justify entering the fault tolerant quantum computing (FTQC) regime? Do we require a quantum supremacy experiment on a FTQC such as the one demonstrated by the Google-led collaboration or does a more appropriate alternative exist?

Let's say that the we answer the latter question in the affirmative and assume 53 qubits is sufficient to realize an equivalent quantum supremacy in the FTQC regime (i.e. 53 logical qubits), can we estimate the transition from NISQ to FTQC to meaningfully occur once a system capable of error correcting the $n$ number of noisy qubits with single qubit¹ error rate $\epsilon$ to yield 53 logical qubits capable of completing a similar RCS task with XEB benchmarking? If so, what do we estimate the order of $n$ noisy qubits and $\epsilon$ error rate(s) to be?

Another way to think about this: What would an update to this 2018 graph from Google AI Blog look like?

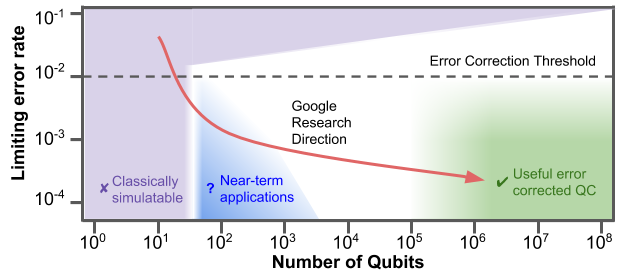
Any answers which explain the current state of thinking with respect to the question would be fantastic.

---

_{1. Setting aside that single qubit errors are far from the only errors we're concerned with.}

Answer 1

The most rigorous definition of NISQ that I've come across resides in The Complexity of NISQ, where the authors define the NISQ complexity class. This class contains a set of problems solvable by a BPP machine with access to a device that only has noisy quantum gates, noisy state preparation at the start and noisy measurements at the end of the program. The prevention of mid-circuit measurements or reset gates prevent this device from doing FTQC.

The authors were then able to prove that NISQ is weaker than BQP and stronger than BPP via oracle separations. They're lots of complexity-theoretical kinks that need to be worked out with this definition, but it's the best we have as far as I'm aware. Notice that specific qubit counts or error rates are not mentioned.

Regarding your "unpacking" questions, trying to get a handle on the comparisons of physical and logical qubits, comparisons which are descendants of discussions about NISQ vs FTQC, can get messy. Starting from the application and replacing the physical vs logical qubit distinction with number of qubits at a certain error rate is neater.

Let's consider the problem RCS is trying to solve. RCS is typically used to demonstrate that a quantum computer can do some task better than existing classical computers. We haven't needed error correction to accomplish this. Recent experiments from Google e.t al. and Quantinuum e.t al is enough evidence.

Now, if we were trying to solve a different problem, say, trying to demonstrate the minimum viable application of some scientific interest better than existing classical computers, that could be, for example, simulating the 2D transverse field Ising model. This will require something on the order of 200 qubits at a $10^{-11}$ error rate. The resource requirements for this application will go down significantly, but it's unlikely that they will be low enough for us to get away from performing QEC in order to run it.