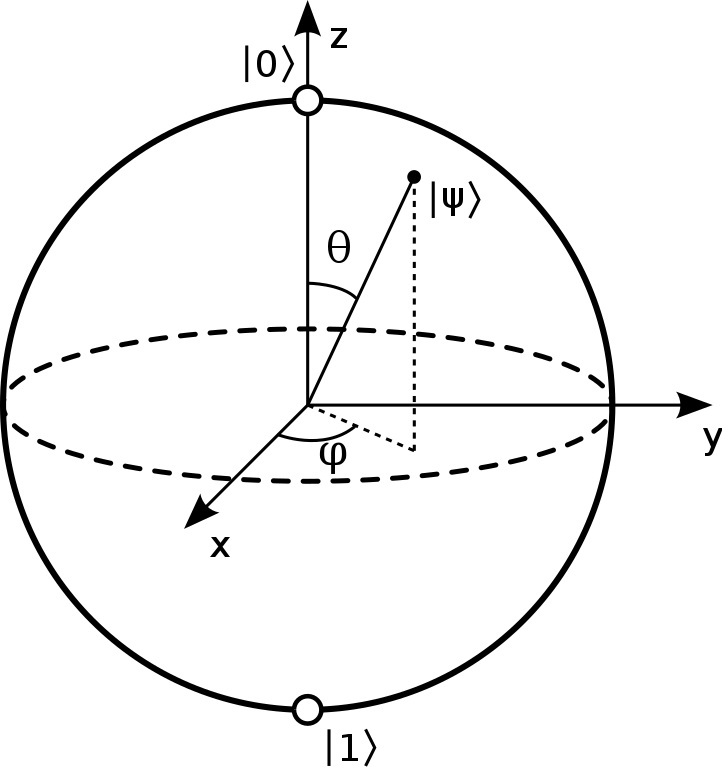
To follow up on hizqial's answer, these two spheres represent two different things.
- The Bloch sphere is a way to visualize the state of a single qubit in every way possible. On the image below you see that the North Pole of the sphere represents the $|0\rangle$ state, whereas the South Pole represents the $|1\rangle$ state. Every point between the two represents a superposition with relative phase $\phi$ and amplitude angle $\theta$. So every state can be represented as $|\psi\rangle = cos(\frac{\theta}{2})|0\rangle + e^{I\phi}sin(\frac{\theta}{2})|1\rangle$, as seen on the image below :
- The Q-sphere is different. It is there to represent transformations between different multi-qubits states, until 5 qubits. The North Pole of the Q-sphere represents the $|0\cdots 0\rangle$ state and the South Pole represents the $|1\cdots 1\rangle$ state. Then the other states are put between them with the ones with more 1s are put closer to the South Pole. The latitudes is defined as the Hamming Distance. The vector on the sphere represents the state vector of the system, the same way as the Bloch sphere. The phase is not represented physically as with the Bloch sphere, but with a color code. You can see that on the image below, which is an example for a 4-qubit system :

Here, I hope this can help, these are both visualization tools, to gain intuition about quantum systems.