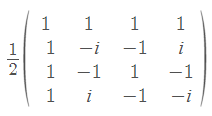I am not sure how to find the following matrix (the inverse of Quantum Fourier Transform) in terms of elementary quantum gates? I am using Qiskit to implement it.
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
Read the circuit for the QFT on 2 qubits and reverse and dagger everything
$$ (1 \otimes H)(Controlled(2,S_1^\dagger))(H \otimes 1) $$
Edit:
For how: Implementation of inverse QFT?
This decomposition is $m=2$ on https://en.wikipedia.org/wiki/Quantum_Fourier_transform Note that $R_2 = S$
$1 \otimes H$ to denote Hadamard on the second qubit. $H \otimes 1$ for Hadamard on the first. $Controlled(2,S_1^\dagger)$ for use qubit 2 as the control and assuming that apply $S^\dagger$ on the first qubit.
-
$\begingroup$ I am not sure I understand the notation. By (1⊗H), do you mean applying a Hadamard gate to the first qubit? And I am not sure what (Controlled(2,S1)) means? Also, could you explain how you came up with this? Thank you in advance. $\endgroup$– MaxxCommented Jul 12, 2019 at 20:03
-
$\begingroup$ This might help you youtu.be/uuBgK44JrnA $\endgroup$– AmanCommented Jul 15, 2019 at 7:08
-
$\begingroup$ Thank you! I have one last question, how can I represent the controlled-S† gate? I can only find a single-qubit S† gate. $\endgroup$– MaxxCommented Jul 15, 2019 at 16:18
-
$\begingroup$ if you're implementing using QISKit the video shows the code also. $\endgroup$– AmanCommented Jul 16, 2019 at 8:05