See the paper "time optional-optimal quantum computation" and "Flexible layout of surface code computations using AutoCCZ states".
For Clifford operations, time is not a concern at all. Because of gate teleportation and the ability to backdate Pauli corrections in stabilizer circuits, Clifford operations can be precomputed in parallel elsewhere in the machine. At large scales it makes more sense to think of Clifford operations as having a spacetime cost, rather than a time cost. They will occupy some amount of qubits for some amount of time, but those qubits never have to be important ones near a computational bottleneck.
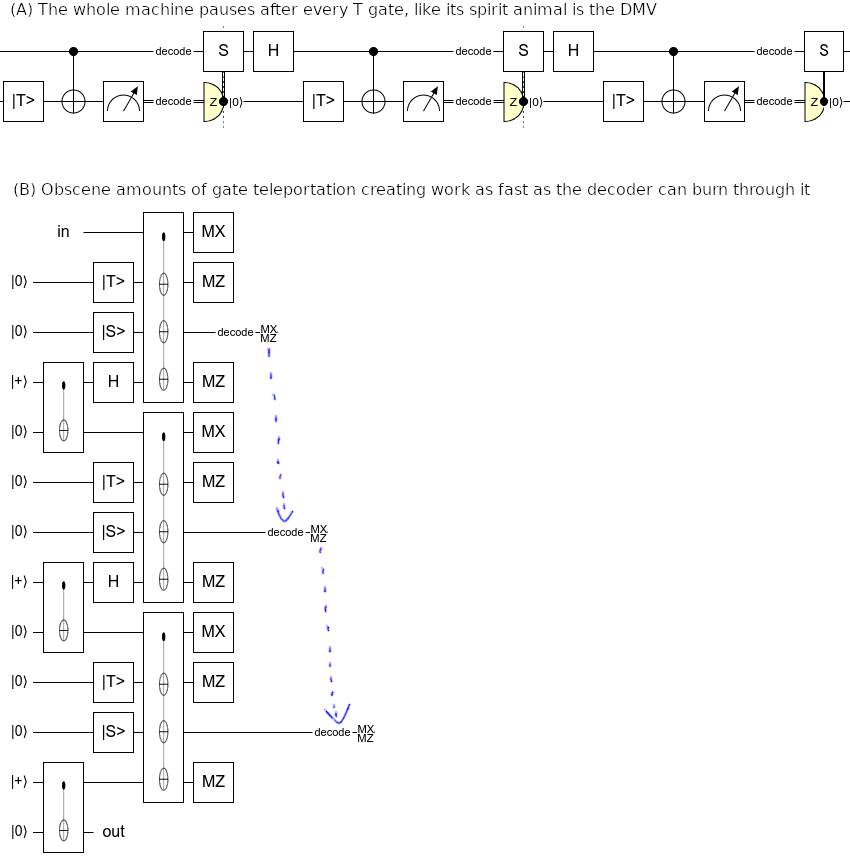
For non-Clifford operations, what matters is linear chains where each gate doesn't commute with the previous one in the chain. Each step in the chain requires a measurement to be decoded, to inform a decision about what to do next somewhere in the quantum computer, to get the next measurement in the sequence. There's no known way to resolve a step of the chain faster than you can run that loop. Doing quantum computation limited by this loop is doing "reaction limited" quantum computation.
At large scales, quantum computations optimized for time don't look like (A) they look like (B):
So it's all about how fast can you do an X-vs-Z measurement, and how fast can your decoder error correct the result. There's no error correction "cycle" on the critical path, because you can arrange things so no two qubit gates are in the critical part of the reaction loop.
That said, running reaction limited requires a lot of magic state factories (for superconducting qubits, it's like 20-ish covering millions of physical qubits total). When fault tolerant quantum computing first starts working, space will be too much of a constraint to have that many factories. And so the rate at which you can do non-Clifford gates will initially be determined by the inability to have enough factories rather than the dependencies between non-Clifford gates. Initially you will see computational styles more similar to the ones proposed in "A Game of Surface Codes".