YouYour approach is correct, but you are taking the partial trace wrong:
$$\rho_A=\text{Tr}_B\big( \rho \big) = \sum_{i} \langle i_B | \rho |i_B \rangle = \langle 0_B | \rho |0_B \rangle + \langle 1_B | \rho |1_B \rangle$$ $$=\frac{1}{2}|0_A \rangle \langle 0_A | + \frac{1}{2}|0_A \rangle \langle 0_A | = |0_A \rangle \langle 0_A | $$
Clearly you can see now: $\rho_A^2=\rho_A$ and so the subsystem is pure, therefore the composite system is unentangled.
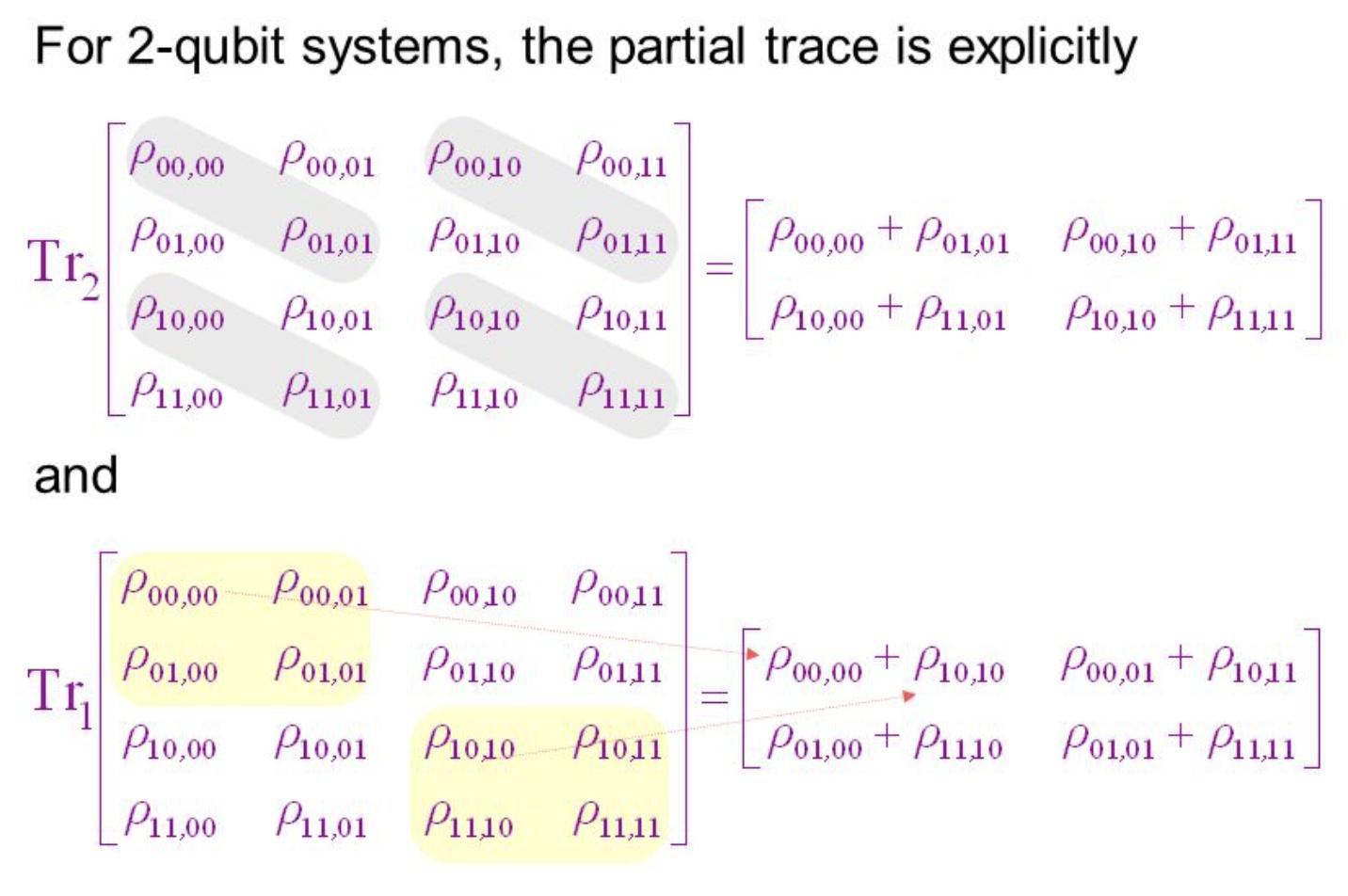
A really easy way to remember partial traces in the computational basis for 2-bit systems is the following:
This picture is not mine, it is from Prof. Michele Mosca's Lecture Notes at University of Waterloo; publicly available.