Understanding the classification of quantum states based on partial transposition: representations of the bipartite density matrix

Question

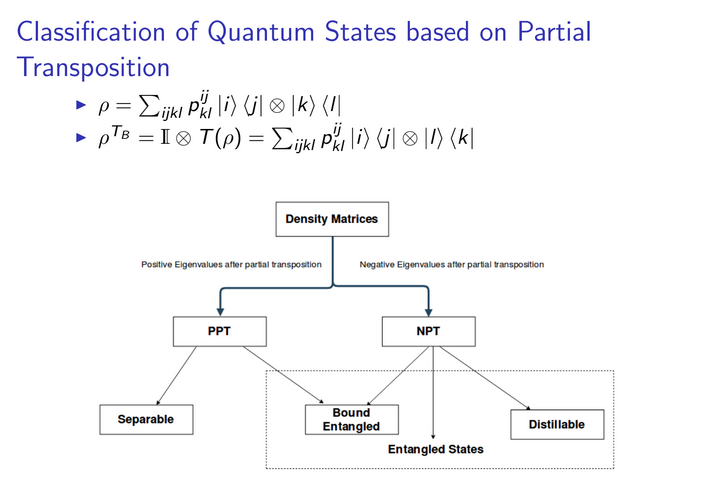
I'm going through some slides on the PPT/NPT criteria along with Horodecki's paper, and I'm kind of stuck. Let's take this slide:

Firstly, why can we write a bipartite density matrix as $\sum_{ijkl}\rho_{kl}^{ij}|i\rangle\langle j|\otimes |k\rangle\langle l|$? What states are the index labels $i, j, k, l$ referring to?

Secondly, Horodecki says on page 21 that if a $\rho_{AB}$ is separable then the new matrix $\rho_{AB}^{T_B}$ with matrix elements can be defined in some product basis as $\langle m|\langle \mu |\rho_{AB}^{T_B}|n\rangle|\nu\rangle = \langle m|\langle \nu|\rho_{AB}|n\rangle|\mu\rangle$. I don't quite understand where they're getting this form from. What are $|m\rangle, |n\rangle, |\mu\rangle, |\nu\rangle$?

Answer 1

For any orthonormal basis that you pick, call it $|e_i\rangle$, you can write a matrix in terms of that basis as $$ \rho=\sum_{i,j}\rho_{i,j}|e_i\rangle\langle e_j|. $$ When you're talking about a bipartite system, a sensible basis is one based on product states, usually the tensor product between two single-system orthonormal bases. So, you might write $$ |e_i\rangle=|n\rangle|\nu\rangle, $$ splitting the sum over $i$ into two sums, over $n$ and $\nu$. Hence, you can write $$ \rho=\sum_{n,\nu,m,\mu}\rho_{n,\nu,m,\mu}|n\rangle\langle m|\otimes|\nu\rangle\langle\mu|. $$ It just happens that they've chosen to change where the different indices are put.

As for the partial transpose, that is simply the definition of the partial transpose. It may not seem so familiar, but recall with the transpose does, $$ \langle e_i|\rho|e_j\rangle=\langle e_j|\rho^T|e_i\rangle. $$ All the partial transpose does is that it only switches the left and right indices for the second subsystem, not the first.

In fact, this definition of $$ \langle n,\nu|\rho|m,\mu\rangle=\langle n,\mu|\rho^{T_B}|m,\nu\rangle $$ is a general definition. It's not about whether $\rho$ is separable or not. The point is that if it is separable, then $\rho^{T_B}$ is still a valid quantum state. On the other hand, if $\rho$ were not separable, there is no reason why $\rho^{T_B}$ need be a valid quantum state. In particular, it could have negative eigenvalues.