I'm researching SPDC's efficacy for use in an optical quantum computing model and I've been trying to figure out exactly what state the photons are in when they come out (as represented by a vector, for example), if I'm using type 1 SPDC and I'm looking at the polarization of the photons.
Please provide any references used =)
First of all, I'll use $\lvert H\rangle$ as a horizontally polarised state and $\lvert V\rangle$ as a vertically polarised state1. There are three modes of light involved in the system: pump (p), taken to be a coherent light source (a laser); as well as signal and idler (s/i), the two generated photons
The Hamiltonian for SPDC is given by $H = \hbar g\left(a^{\dagger}_sa^{\dagger}_ia_p + a^{\dagger}_pa_ia_s\right)$, where g is a coupling constant dependent on the $\chi^{\left(2\right)}$ nonlinearity of the crystal and $a\left(a^{\dagger}\right)$ is the annihilation (creation) operator. That is, there is a possibility of a pump photon getting annihilated and generating two photons2 as well as a possibility of the reverse.
The phase matching conditions for frequencies, $\omega_p = \omega_s + \omega_i$ and wave vectors, $\mathbf{k}_p = \mathbf{k}_s + \mathbf{k}_i$ must also be satisfied.
This is where the two generated (s and i) photons have parallel polarisations, perpendicular to the polarisation of the pump, which can only be used to perform SPDC when the pump is polarised along the extraordinary axis of the crystal.
This means that defining the extraordinary axis as the vertical (horizontal) direction and inputting coherent light along that axis will generate pairs of photons in the state $\lvert HH\rangle\, \left(\lvert VV\rangle\right)$. This is not of much use, so to generate an entangled pair of photons, two crystals are placed next to each other, with extraordinary axes in orthogonal directions. The coherent source is then input with a polarisation of $45^\circ$ to this, such that if the first crystal has an extraordinary axis along the vertical (horizontal) direction, there is a probability of generating photons in the state $\lvert HH\rangle\, \left(\lvert VV\rangle\right)$ as before from the first crystal, as well as a probability of generating photons in the state $\lvert VV\rangle\, \left(\lvert HH\rangle\right)$ from the second crystal.
However, as the light from the pump is travelling through a material, it will also acquire a phase in the first crystal, such that the final state is $$\lvert\psi\rangle = \frac{1}{\sqrt{2}}\left(\lvert HH\rangle + e^{i\phi}\lvert VV\rangle\right).$$
Due to the phase matching conditions, the emitted photon pairs will be emitted at opposite points on a cone, as shown below in Figure 1.
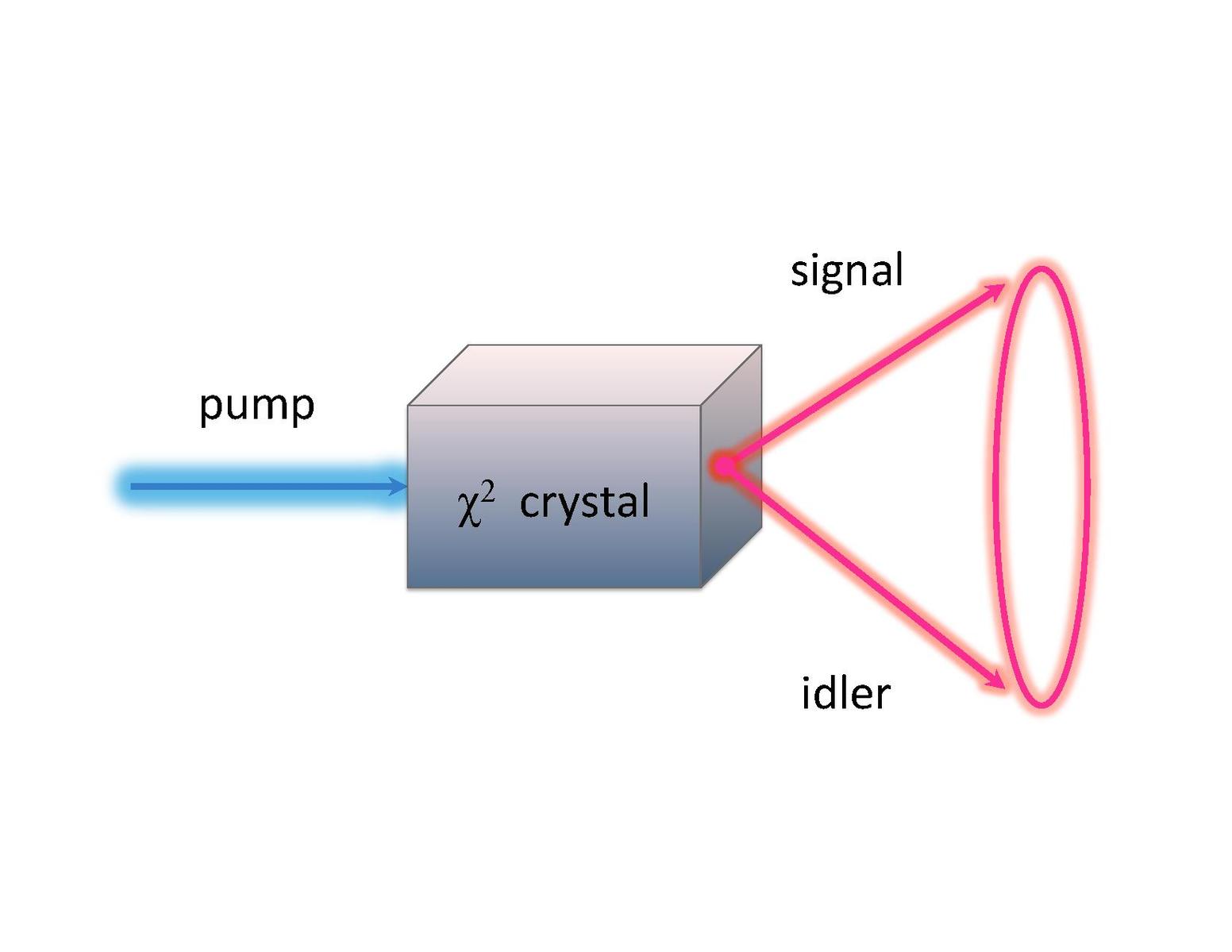Figure 1: A laser beam is input into two type 1 SPDC crystals, with orthogonal extraordinary axes. This results in a probability of emitting a pair of entangled photons at opposite points on a cone. Image taken from Wikipedia.
1 This can be mapped to qubit states using e.g. $\lvert H\rangle = \lvert 0\rangle$ and $\lvert V\rangle = \lvert 1\rangle$
2 called signal and idler for historical reasons
References:
Keiichi Edamatsu 2007 Jpn. J. Appl. Phys. 46 7175
Kwiat, P.G., Waks, E., White, A.G., Appelbaum, I. and Eberhard, P.H., 1999. Physical Review A, 60(2) - and the arXiv version
The existing answer does a good job at describing the state that comes from a SPDC configuration at low conversion efficiency, but it's also worth noting that the single-photon behaviour is not all there is to the process. Thus, in particular, if your conversion efficiency (or you detection time / efficiency / SNR) is good enough that you can detect (and discriminate) the emission of multiple photons in the same mode, then those two-photon events also share quantum correlations between the two modes, as do all higher orders of the photon-statistics distribution.
To be more specific (and ignoring all the polarization, momentum, and phase-matching problems already alluded to by Mithrandir), the light that comes out of a Type II SPDC source in the signal and idler ports is in a two-mode squeezed state
\begin{align} |{\text{TMSV}}\rangle & =S_{2}(\zeta )|0\rangle =\exp(\zeta ^{*}{\hat {a}}{\hat {b}}-\zeta {\hat {a}}^{\dagger }{\hat {b}}^{\dagger })|0\rangle \\& ={\frac {1}{\cosh r}}\sum _{n=0}^{\infty }(\tanh r)^{n}|nn\rangle \\ & \approx \operatorname{sech}(r)\left[|00\rangle + \tanh(r)|11\rangle + \tanh^2(r)|22\rangle + \tanh^3(r) |33\rangle + \cdots \right], \end{align} i.e. just as a detection of a single photon on the signal port is completely (and coherently) correlated with a single photon on the idler port, so too does the observation of a two-photon signal state imply that the idler's mode has been collapsed onto a two-photon state.
Generally, the folks running SPDC setups as single-photon sources run them in a regime where $\tanh(r)$ is small (so most of the time you get vacuum, except when you do get a click on the signal that guarantees a single photon's presence in the idler mode) in order to eliminate the contribution of the higher-order photon-statistics channels, but they're still there, they can be important, and if you don't control appropriately for them then they might overwhelm the single-photon component of your signal.
I should also say that the details change from configuration to configuration (so e.g. Type I SPDC only produces single-mode squeezed vacua, if I understand correctly) but the higher-order terms will generally always occur.
