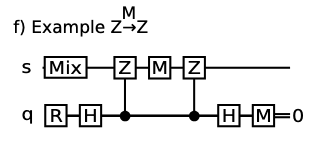
I am reading https://arxiv.org/pdf/2302.02192 by Gidney and it shows how to construct stabilizer flow diagrams. See the following diagram (figure from Appendix A)
The claim here is that qubit s is prepared in the maximally mixed state while qubit q is prepared in $\vert 0\rangle$. One then applies the Hadamard, control Z and measurement in Z basis gates. The measurement gate on qubit s leaves a post-measurement eigenstate but the measurement on q destroys the qubit and only outputs classical information. The claim is that for this circuit, the classical measurement outcome is always 0.
Why is this true? The first control Z gate does nothing since its target is already maximally mixed. The measurement M on qubit s results in the state
$$(\vert 0\rangle + \vert 1\rangle)\vert r\rangle,$$
where $r$ is the outcome of the measurement. Applying another controlled Z gate, we have a Bell state that depends on $r$. Then one can apply the Hadamard on qubit q but this does not guarantee the second measurement has outcome $0$.
How should one read this diagram?