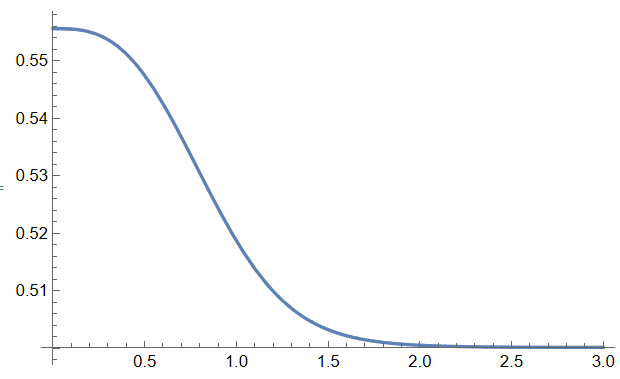
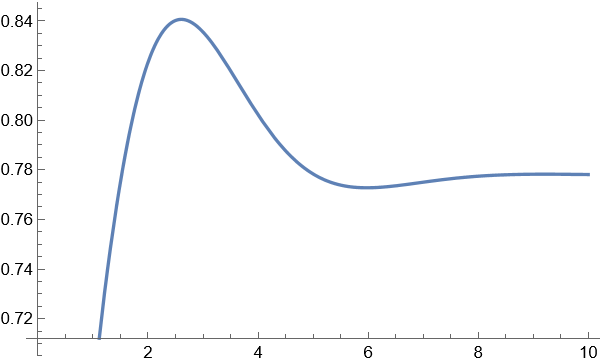
Is the purity $\mathrm{Tr}[\rho(t)^2]$ of a quantum state $\rho(t)$ that evolves according to a time-independent Lindbladian $\partial_t \rho = \mathcal{L}[\rho]$ convex in time $t$?
I suspect the the answer is "no" in general, but I wonder whether there are any useful characterizations of the kinds of Lindbladians which lead to purities that are convex in time. The furthest I have gotten on my own is a sufficient condition which I suspect is not a necessary condition: if the map $\mathcal{L}^\dagger \mathcal{L} - \mathcal{L}\mathcal{L}^\dagger$ is positive ($\mathcal{L}^\dagger$ is the adjoint of $\mathcal{L}$), then the purity is convex in time. I arrived at this condition by expressing the second time derivative of purity as $\partial_t^2 \mathrm{Tr}[\rho(t)^2] = \mathrm{Tr}[\rho(t) ((\mathcal{L} + \mathcal{L}^\dagger)^2 + \mathcal{L}^\dagger \mathcal{L} - \mathcal{L} \mathcal{L}^\dagger)[\rho(t)]]$.
I'd also be interested in similar characterizations for any entanglement monotones of the form $\mathrm{Tr}[f(\rho(t))]$, where $f$ is a convex function.
Thanks!
Edit: this is a cross post from https://math.stackexchange.com/questions/4927440/are-there-any-useful-convexity-properties-of-quantum-dynamical-semigroups.