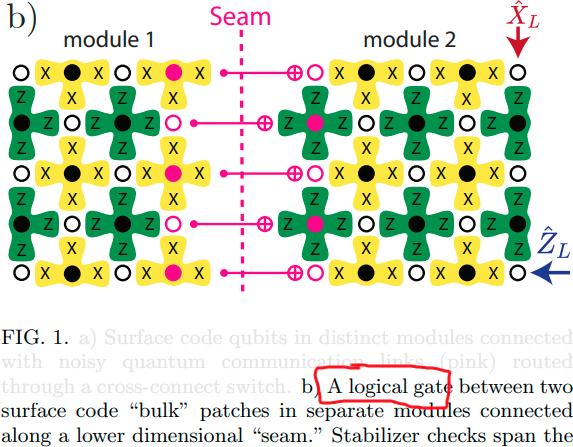
According to https://arxiv.org/abs/1111.4022, to perform a logical CNOT using lattice surgery, three patches of surface codes are needed and nearest neighbour operations are performed. However, I recently come across a paper https://arxiv.org/pdf/2302.01296. In Figure 1(b) it's claiming that using two patches and CNOT gates between boundary qubits we can perform a logical CNOT gate. What's the premise of this operation? Or perhaps I'm misunderstanding something?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
According to https://arxiv.org/abs/1111.4022, to perform a logical CNOT using lattice surgery, three patches of surface codes are needed
They show that three patches are sufficient, not that three patches are needed. You can avoid the third patch, at least in a marginal cost sense, by e.g. doing the CNOT while routing one logical qubit past the other.
In Figure 1(b) it's claiming that using two patches and CNOT gates between boundary qubits we can perform a logical CNOT gate.
The caption says "A logical gate", not "A logical CNOT". The logical gate they are doing is $M_{XX}$.
-
$\begingroup$ Thanks. Yes that's how I guessed it was, but not sure which logical gate it corresponds to. Would you mind providing the reference for the logical CNOT? I mean, "doing CNOT while routing one logical qubit past the other". $\endgroup$ Commented May 20 at 1:03