Two Quantum State Density Matrices with Unequal Off-Diagonal Elements but Equal Magnitudes

Question

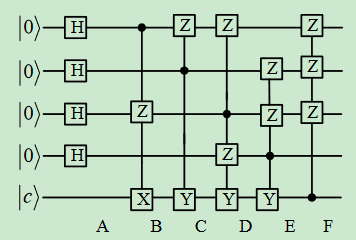
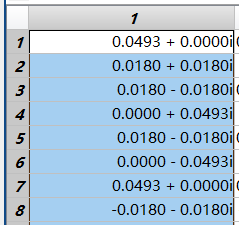
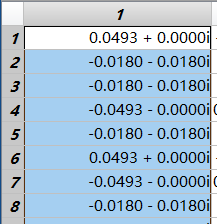
I recently read Kitaev's paper on magic state distillation. I want to verify whether the circuit for the [5,1,3] encoder can map a single qubit state T_0 to the logical state T_1 in the encoded space. I denote the density matrix of the output state of the quantum circuit as A, and the density matrix of the logical state T_1 in the encoded space as B. The result shows that A and B have completely identical diagonal elements, and the corresponding off-diagonal elements of A and B are different but have equal magnitudes. How should we interpret this result? Can I consider that the single qubit state T_0 has been successfully mapped to the logical state T_1 in the encoded space?Thank you very much Below are the quantum circuits, density matrices A and B, and their respective local elements.

Answer 1

Generally speaking, two density matrices with equal diagonal correspond to states which are indistinguishable when measured in the computational basis, but which result in different outcome probabilities with respect to some other measurement basis. Having off-diagonal elements that are equal in absolute value but not in phase doesn't significantly affect this. Consider as a simple example two single-qubit states like $$\begin{pmatrix}1/2 & 1/2 \\ 1/2 & 1/2\end{pmatrix}, \qquad \begin{pmatrix}1/2 & i/2 \\ -i/2 & 1/2\end{pmatrix}.$$ These look identical in the $Z$ basis, both giving outcome probabilities $p_0=p_1=1/2$, but in the $X$ basis the first one gives probabilities $p_+=1$, $p_-=0$ while the second one gives $p_+=p_-=1/2$.