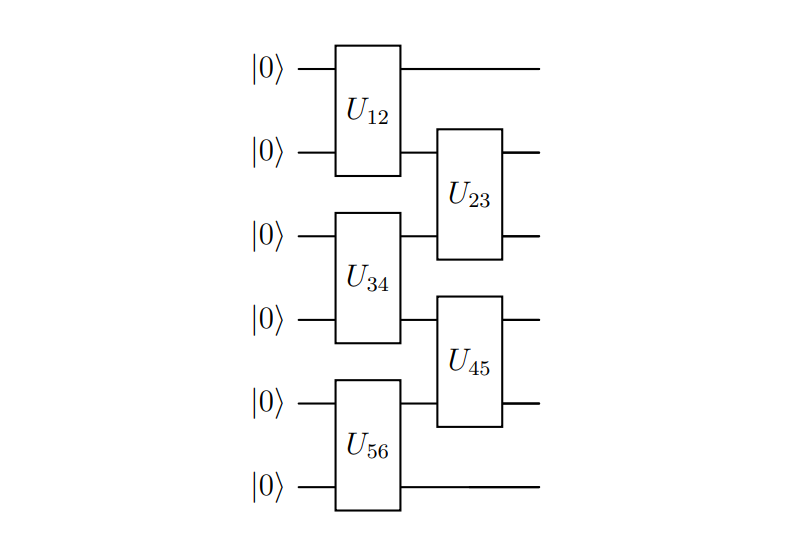
Consider the random quantum circuit below where the gates are randomly taken from SU(4) accordingly with the Haar measure. I am looking to determine an upper bound on the entanglement entropy between the first 3 qubits and the last 3 qubits. How can I rigorously do so?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
This looks like a homework question, so I give hints in the form of useful facts.
The entanglement between the partition of the first three qubits and the last three qubits only occurs due to the gate $U_{34}$.
Also, entanglement is a global property of a quantum state, and it is invariant under local unitary transformations. What would be the local unitary transformations with respect to the partition that we are interested in?
The maximum entanglement entropy can be determined by looking at the dimension of one of the partitions. This is because the reduced density matrix of one of the partitions can be a maximally mixed state. What is the entropy of that?
