Converting $H$ gate to $R_x$ and $R_z$

Question

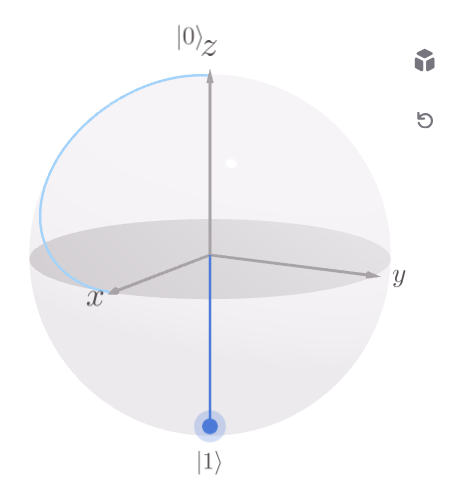
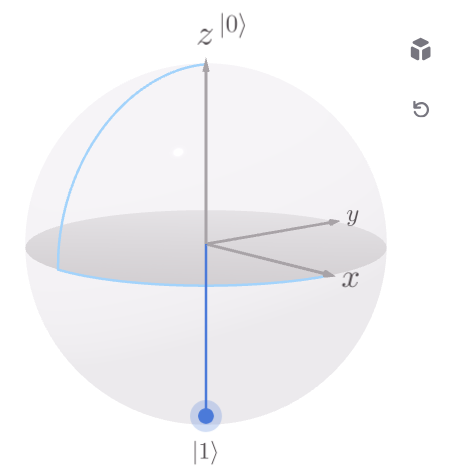
EDIT: My solution is supposed to work for $|1\rangle$ state too. See https://imgur.com/a/7F1cHu4

Right of the bat the answer is $$H=R_z(\pi/2)R_x(\pi/2)R_z(\pi/2)\,.$$ My question is, I cannot reach the same answer using the Bloch sphere.

Clearly, $H$ transform $|0\rangle$ to the position below.

One would think that he can do a $R_x(\pi/2)R_z(\pi/2)$ to get the same result. However

$$ \begin{align} R_x(\pi/2)R_z(\pi/2)&=\begin{pmatrix} \cos\left(\pi/4\right) & -i\sin\left(\pi/4\right) \\ -i\sin\left(\pi/4\right) & \cos\left(\pi/4\right) \end{pmatrix}\begin{pmatrix} e^{-i\frac{\pi}{4}} & 0 \\ 0 & e^{i\frac{\pi}{4}} \end{pmatrix}\\ &=\begin{pmatrix}\frac{1}{2}-i\frac{1}{4}&\frac{1}{}-i\frac{1}{2}\\-\frac{1}{2}-i\frac{1}{2}&\frac{1}{2}+i\frac{1}{2}\end{pmatrix} \end{align} $$

The visualization also confirms this

What mistake have I made?

Answer 1

There's about three little niggles here. First, order of calculation. If you want to get rid of the first $Z$ rotation from your calculation, then that is the right-most one in the product, not the left-most one.

Next, your multiplication of the matrices seems a bit dodgy. You want $$ \frac{1}{\sqrt{2}}\begin{bmatrix} e^{-i\pi/4} & 0 \\ 0 & e^{i\pi/4} \end{bmatrix}\begin{bmatrix} 1 & -i \\ -i & 1 \end{bmatrix} =\frac{e^{-i\pi/4}}{\sqrt{2}}\begin{bmatrix} 1 & -i \\1 & i \end{bmatrix}. $$

Finally, maybe you're thinking "this still isn't Hadamard". But it isn't supposed to be Hadamard. It's only supposed to be equivalent to Hadamard when acting on the $|0\rangle$ state. So look at the first column. This describes the output that you're expecting.