How to perform a state density modification for a single targeted state only?

Question

I have a question about single target state modification... Suppose we have a 3 qubit state density distribution as follows (prenormalized):

$$\begin{bmatrix} |000\rangle & 3 \\ |001\rangle & 4 \\ |010\rangle & 5 \\ |011\rangle & 6 \\ |100\rangle & 7 \\ |101\rangle & 8 \\ |110\rangle & 9 \\ |111\rangle & 10 \\ \end{bmatrix}$$

and we would like to do a "whack-a-mole" and whack $|010\rangle$ down to $0$ while keeping all the other proportions unchanged, i.e.

$$\begin{bmatrix} |000\rangle & 3 \\ |001\rangle & 4 \\ |010\rangle & \color{red}0 \\ |011\rangle & 6 \\ |100\rangle & 7 \\ |101\rangle & 8 \\ |110\rangle & 9 \\ |111\rangle & 10 \\ \end{bmatrix}$$

How might one do this using physically allowed quantum state manipulations?

Answer 1

You're using some unusual notation and terminology that don't entirely fit. I'm assuming you question is that you are starting from a state $$ |\psi\rangle=3|000\rangle+4|001\rangle+5|010\rangle+6|011\rangle+7|100\rangle+8|101\rangle+9|110\rangle+10|111\rangle)/\sqrt{380} $$ and you want to convert it into $$ |\phi\rangle=3|000\rangle+4|001\rangle+6|011\rangle+7|100\rangle+8|101\rangle+9|110\rangle+10|111\rangle)/\sqrt{355}. $$ You have a vast range of options for how you might do this. By far the simplest is essentially the suggestion of TristanNemoz where you run a probabilistic protocol. In terms of a quantum circuit, this looks like

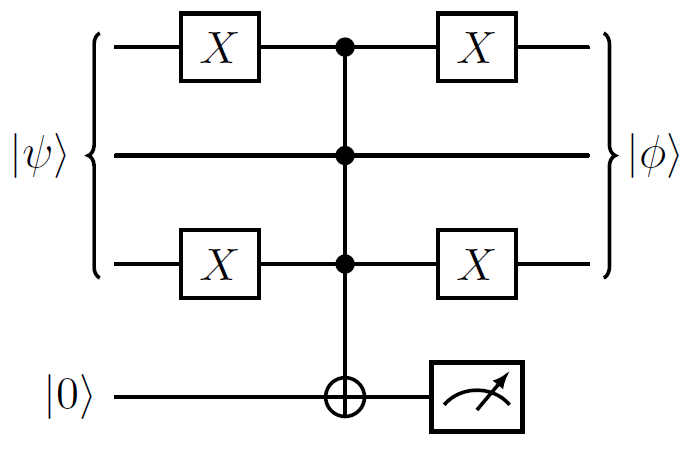
and works provided the measurement gives the answer 0, which happens with probability $\frac{355}{380}=\frac{71}{76}$. The circuit is simple, and has the further advantage that you don't actually need to know what state $|\psi\rangle$ is, only the term that you want to knock out. The disadvantage, of course, is that it is probabilistic.

There certainly exist deterministic options, assuming that you know both $|\psi\rangle$ and $|\phi\rangle$. You just need to choose any unitary that satisfies $$ U|\psi\rangle=|\phi\rangle. $$ You have to complete its action on an orthonormal basis that includes $|\psi\rangle$, with the choice of what the corresponding output states are giving you a lot of freedom to potentially alter how easy/hard the unitary is to construct. Here is one such example (which I have no desire to decompose into gates!): $$ \left( \begin{array}{cccccccc} \frac{110}{113}+\frac{12 \sqrt{\frac{3}{13871767}}}{5}+\frac{9}{10 \sqrt{1349}} & \frac{2 \left(339-2 \sqrt{30849}\right)}{565 \sqrt{1349}} & 4 \sqrt{\frac{3}{13871767}}+\frac{3}{2 \sqrt{1349}} & -\frac{6}{113}+\frac{24 \sqrt{\frac{3}{13871767}}}{5}+\frac{9}{5 \sqrt{1349}} & -\frac{7}{113}+\frac{4 \sqrt{\frac{21}{1981681}}}{5}+\frac{21}{10 \sqrt{1349}} & -\frac{8}{113}+\frac{32 \sqrt{\frac{3}{13871767}}}{5}+\frac{12}{5 \sqrt{1349}} & -\frac{9}{113}+\frac{36 \sqrt{\frac{3}{13871767}}}{5}+\frac{27}{10 \sqrt{1349}} & -\frac{10}{113}+8 \sqrt{\frac{3}{13871767}}+\frac{3}{\sqrt{1349}} \\ \frac{15}{\sqrt{122759 \left(703+4 \sqrt{30849}\right)}} & \frac{8+\sqrt{30849}}{5 \sqrt{1349}} & -\frac{\sqrt{30849}-182}{91 \sqrt{1349}} & \frac{30}{\sqrt{122759 \left(703+4 \sqrt{30849}\right)}} & -\frac{\sqrt{30849}-182}{65 \sqrt{1349}} & \frac{40}{\sqrt{122759 \left(703+4 \sqrt{30849}\right)}} & \frac{45}{\sqrt{122759 \left(703+4 \sqrt{30849}\right)}} & -\frac{2 \left(\sqrt{30849}-182\right)}{91 \sqrt{1349}} \\ -\frac{5 \sqrt{\frac{3}{10283}}}{2} & 0 & \frac{\sqrt{\frac{339}{91}}}{2} & -5 \sqrt{\frac{3}{10283}} & -\frac{5 \sqrt{\frac{7}{4407}}}{2} & -\frac{20}{\sqrt{30849}} & -\frac{15 \sqrt{\frac{3}{10283}}}{2} & -\frac{25}{\sqrt{30849}} \\ -\frac{6}{113}+\frac{24 \sqrt{\frac{3}{13871767}}}{5}+\frac{9}{5 \sqrt{1349}} & \frac{4 \left(339-2 \sqrt{30849}\right)}{565 \sqrt{1349}} & 8 \sqrt{\frac{3}{13871767}}+\frac{3}{\sqrt{1349}} & \frac{101}{113}+\frac{48 \sqrt{\frac{3}{13871767}}}{5}+\frac{18}{5 \sqrt{1349}} & -\frac{14}{113}+\frac{8 \sqrt{\frac{21}{1981681}}}{5}+\frac{21}{5 \sqrt{1349}} & -\frac{16}{113}+\frac{64 \sqrt{\frac{3}{13871767}}}{5}+\frac{24}{5 \sqrt{1349}} & -\frac{18}{113}+\frac{72 \sqrt{\frac{3}{13871767}}}{5}+\frac{27}{5 \sqrt{1349}} & -\frac{20}{113}+16 \sqrt{\frac{3}{13871767}}+\frac{6}{\sqrt{1349}} \\ -\frac{7}{113}+\frac{4 \sqrt{\frac{21}{1981681}}}{5}+\frac{21}{10 \sqrt{1349}} & -\frac{70}{\sqrt{457311 \left(703+4 \sqrt{30849}\right)}} & 4 \sqrt{\frac{7}{5945043}}+\frac{7}{2 \sqrt{1349}} & -\frac{14}{113}+\frac{8 \sqrt{\frac{21}{1981681}}}{5}+\frac{21}{5 \sqrt{1349}} & \frac{290}{339}+\frac{28 \sqrt{\frac{7}{5945043}}}{5}+\frac{49}{10 \sqrt{1349}} & -\frac{56}{339}+\frac{32 \sqrt{\frac{7}{5945043}}}{5}+\frac{28}{5 \sqrt{1349}} & -\frac{21}{113}+\frac{12 \sqrt{\frac{21}{1981681}}}{5}+\frac{63}{10 \sqrt{1349}} & -\frac{70}{339}+8 \sqrt{\frac{7}{5945043}}+\frac{7}{\sqrt{1349}} \\ -\frac{8}{113}+\frac{32 \sqrt{\frac{3}{13871767}}}{5}+\frac{12}{5 \sqrt{1349}} & -\frac{80}{\sqrt{457311 \left(703+4 \sqrt{30849}\right)}} & \frac{4}{\sqrt{1349}}+\frac{32}{\sqrt{41615301}} & -\frac{16}{113}+\frac{64 \sqrt{\frac{3}{13871767}}}{5}+\frac{24}{5 \sqrt{1349}} & -\frac{56}{339}+\frac{32 \sqrt{\frac{7}{5945043}}}{5}+\frac{28}{5 \sqrt{1349}} & \frac{275}{339}+\frac{32}{5 \sqrt{1349}}+\frac{256}{5 \sqrt{41615301}} & -\frac{24}{113}+\frac{96 \sqrt{\frac{3}{13871767}}}{5}+\frac{36}{5 \sqrt{1349}} & -\frac{80}{339}+\frac{8}{\sqrt{1349}}+\frac{64}{\sqrt{41615301}} \\ -\frac{9}{113}+\frac{36 \sqrt{\frac{3}{13871767}}}{5}+\frac{27}{10 \sqrt{1349}} & \frac{6 \left(339-2 \sqrt{30849}\right)}{565 \sqrt{1349}} & 12 \sqrt{\frac{3}{13871767}}+\frac{9}{2 \sqrt{1349}} & -\frac{18}{113}+\frac{72 \sqrt{\frac{3}{13871767}}}{5}+\frac{27}{5 \sqrt{1349}} & -\frac{21}{113}+\frac{12 \sqrt{\frac{21}{1981681}}}{5}+\frac{63}{10 \sqrt{1349}} & -\frac{24}{113}+\frac{96 \sqrt{\frac{3}{13871767}}}{5}+\frac{36}{5 \sqrt{1349}} & \frac{86}{113}+\frac{108 \sqrt{\frac{3}{13871767}}}{5}+\frac{81}{10 \sqrt{1349}} & -\frac{30}{113}+24 \sqrt{\frac{3}{13871767}}+\frac{9}{\sqrt{1349}} \\ -\frac{10}{113}+8 \sqrt{\frac{3}{13871767}}+\frac{3}{\sqrt{1349}} & \frac{4}{\sqrt{1349}}-8 \sqrt{\frac{91}{457311}} & \frac{5}{\sqrt{1349}}+\frac{40}{\sqrt{41615301}} & -\frac{20}{113}+16 \sqrt{\frac{3}{13871767}}+\frac{6}{\sqrt{1349}} & -\frac{70}{339}+8 \sqrt{\frac{7}{5945043}}+\frac{7}{\sqrt{1349}} & -\frac{80}{339}+\frac{8}{\sqrt{1349}}+\frac{64}{\sqrt{41615301}} & -\frac{30}{113}+24 \sqrt{\frac{3}{13871767}}+\frac{9}{\sqrt{1349}} & \frac{239}{339}+\frac{10}{\sqrt{1349}}+\frac{80}{\sqrt{41615301}} \\ \end{array} \right) $$

Finally, another method that you could consider if you wanted something deterministic, but you didn't know $|\psi\rangle$ is amplitude amplification, but I suspect that's going beyond the scope of what you want.

---

To get the big unitary matrix, I used a piece of Mathematica code:

psi0 = {3, 4, 5, 6, 7, 8, 9, 10}/Sqrt[380];
ns1 = Orthogonalize[NullSpace[{psi0}]];
ns1 = Join[{psi0}, ns1]
phi0 = {3, 4, 0, 6, 7, 8, 9, 10}/Sqrt[355];
ns2 = Orthogonalize[NullSpace[{phi0}]];
ns2 = Join[{phi0}, ns2]
U = FullSimplify[
Plus @@ (Transpose[{#[[2]]}].{#[[1]]} & /@ Transpose[{ns1, ns2}])]

This is actually major overkill. It's good enough to find any unitaries $U_1$ such that $U_1|000\rangle=|\psi_0\rangle$ and $U_1|000\rangle=|\phi_0\rangle$ (which are relatively straightforward to construct) and then just run the circuit $U_1^\dagger U_2$. Indeed, this paper shows (and ultimately gives a construction) for how a unitary can be constructed using no more than 4 controlled-not gates!.

Answer 2

The projectors $\mathcal{M}_0=|010\rangle\!\langle010|$ and $\mathcal{M}_1=I-|010\rangle\!\langle010|$ define a measurement. Applying this measurement, you will get outcome $0$ with probability $\frac{5^2}{3^2+\cdots+10^2}=\frac{5}{76}$ and outcome $1$ with probability $\frac{71}{76}\approx93.42\%$.

If you get outcome $1$, then the resulting state will be the one that you want.