An intuitive definition of "One-way LOCC distance"

Question

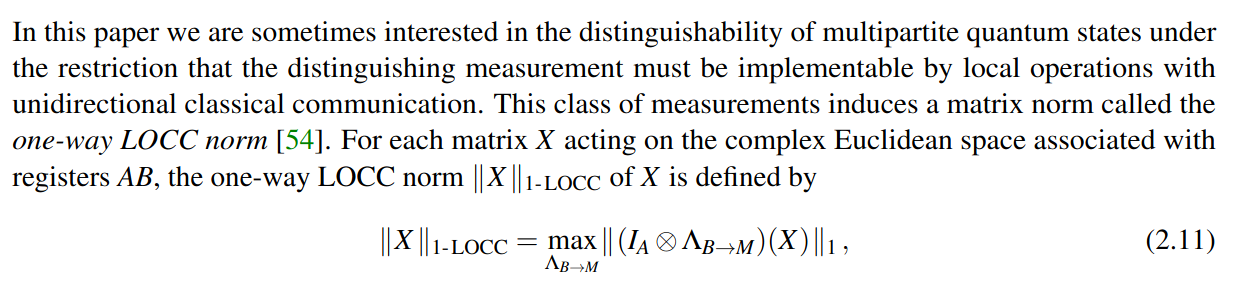
I am reading up on this paper which covers many aspects about fidelity, separability, and came across the "One way LOCC-distance" in section 2.4. Below is an excerpt:

I am having trouble understanding what exactly this norm represents, and how it is used in the rest of the paper.

This $\Lambda_{B \rightarrow M}$ represents a quantum-to-classical channel, but I'm not really getting the intuition of this operation.

Why is this quantum to classical channel having the tensor product acted on it? And how is it even unitary, it performs a measurement and is thus not reversible, right?

Answer 1

$\Lambda_{B\rightarrow M}$ is a channel, so its action on $X$ is that of a superoperator (rather than a unitary matrix). The tensor product indicates that the composite channel acting on $X$ consists of the tensor product of the identity channel acting on subsystem $A$ and $\Lambda_{B\rightarrow M}$ on subsystem $B$. Basically, the norm represents the largest trace norm of $X$ that can be obtained after performing measurements solely on subsystem $B$.