Can we design a circuit that outputs desired estimates?

Question

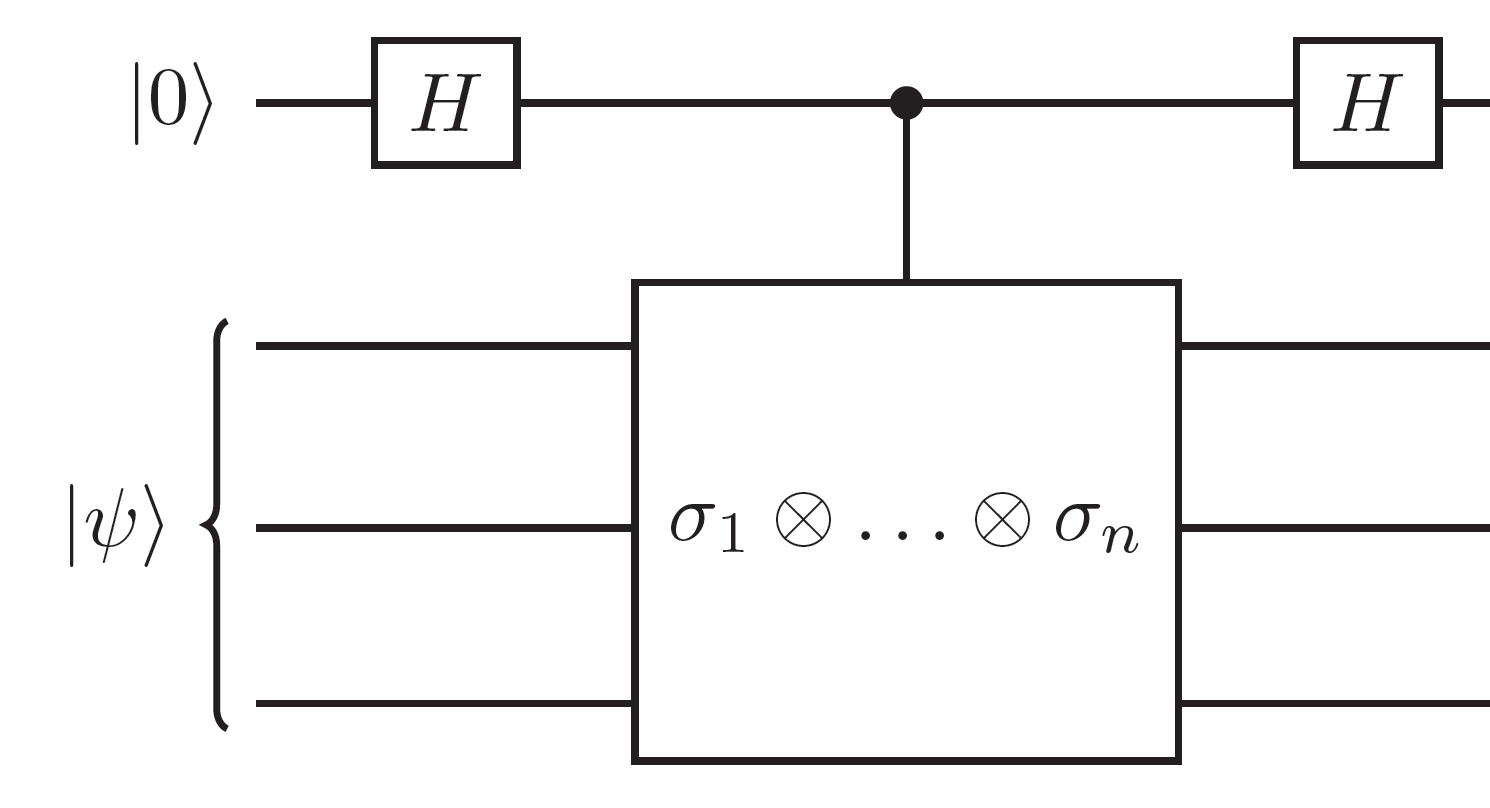
If we have state $\lvert\psi\rangle \in (\mathbb{C}^{2})^{\otimes n}$ in an $\textit{n}$-qubit system with Pauli operators $P$ such that $P \in \{I, X, Y, Z\}^{n}$, how can we design a circuit/procedure using multiple circuits and/or classical processing that would output a real output $\alpha$ which is on average equal to $$ Tr((P_{1} \otimes ... \otimes P_{n})\lvert \psi \rangle \langle \psi \rvert) $$

Maybe as a start, say our possible outcomes are $x \in \{0, 1\}^{n}$, so that when we measure an individual qubit $j$ of $\lvert \psi \rangle$ with projections $\{P_{j, 0}, P_{j, 1}\}$ we get an outcome $x_{i} \in \{0/1\}$. Can we obtain outcomes $x_{1},...,x_{n}$ simultaneously and what is the probability of obtaining those outcomes simultaneously? If I'm understanding observables correctly, the observable for this system would be $$O = \sum\limits_{i \in \{I, X, Y, Z\}^{n}} \sum\limits_{j \in \{0, 1\}} x_{j} P_{i, j}$$ although I'm not 100% sure if that's relevant to the problem.

Answer 1

To get the expectation that you want, you don't need to measure the individual observables. Instead, it would be sufficient to use $$ O=P_1\otimes P_2\otimes\ldots\otimes P_n. $$ You can measure this (which has a single $\pm 1$ outcome) by introducing a single ancilla and applying the circuit where (because I'm lazy and used an image I already had), I've used $\sigma_i$ in place of $P_i$.

If $p_0$ and $p_1$ are the probabilities of getting 0 and 1 outcomes, then $$ \langle\psi|O|\psi\rangle=p_0-p_1=2p_0-1. $$