4
$\begingroup$

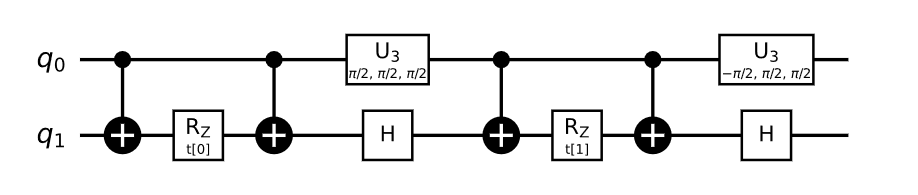
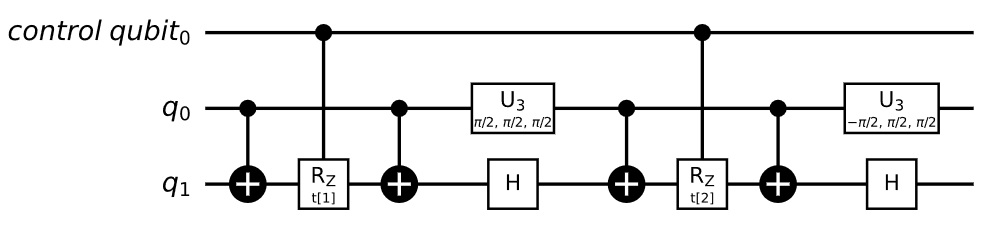
I had a question last week regarding the simulation of Hamiltonians composed of the sum of Pauli products: How can I simulate Hamiltonians composed of Pauli matrices? I'm having a follow-up question: still for those two Hamiltonians: $$ H_{1} = X_1+ Y_2 + Z_1\otimes Z_2 \\ H_{2} = X_1\otimes Y_2 + Z_1\otimes Z_2 $$ How can I perform the 'controlled version' of them? The thing really confused me is the 'tensor product term': for both $H_1$ and $H_2$, the two qubits are coupled, but if I want to do the controlled time-evolution simulation, should I couple the whole thing with the third qubit? If so, how to do that?

Thanks:)

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ The last post you used implicitly used exponentiation - do you want to use that approach or VQEs? (what's the end goal here might be a better question) $\endgroup$
    – C. Kang
    Commented Nov 12, 2020 at 0:23
  • 1
    $\begingroup$ @C. Kang Thanks for the comment! I still prefer the exponentiation approach:) $\endgroup$
    – ZR-
    Commented Nov 12, 2020 at 0:32

1 Answer 1

Reset to default
3
$\begingroup$

So we know that $e^{i t H_2}$ has the following circuit:

enter image description here

From this answer along with page 13 from this paper we can try to build the controlled-version of $e^{i t H_2}$ as follow:

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you so much for the answer! Is there still a global phase gate needed on the control qubit? $\endgroup$
    – ZR-
    Commented Nov 12, 2020 at 14:34
  • 1
    $\begingroup$ The global phase gate is not needed if we don't have $e^{i I t}$ from my understanding. So unless our Hamiltonian is something like $H = XY + ZZ + II$ then you won't be needing it. $\endgroup$
    – KAJ226
    Commented Nov 12, 2020 at 18:14
  • $\begingroup$ Got it. Thanks!! $\endgroup$
    – ZR-
    Commented Nov 12, 2020 at 20:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.