(1) Both filters and attenuators are used
Let me just start by saying that non-attenuating filters have not been completely ruled out by people working in the design of cold quantum computers. I will use quotes from three papers, all from 2018, to support this point.
"These residual cavity photons arise from a variety of sources, e.g. thermal microwave photons from blackbody radiation that is
improperly attenuated or filtered in the cryogenic environment
[12]."
Note that the authors mention that the photons have to be attenuated or filtered.
"Further improvement may be possible with more careful choice of attenuator/filter configuration or active cooling techniques."
Notice that the authors mention both attenuators and filters together here.
"RF lines on the other hand are realized
as semi-rigid microwave cables and contain various microwave components
such as attenuators, filters or amplifiers."
Again the authors do not rule out the use of filters!
"This coupling opens a channel for auxiliary components in the
microwave wiring to affect the temperature of the cavity mode. Examples of such components are commercial cryogenic attenuators, filters, isolators, etc."
Again the authors do not rule out the use of filters, and later they even say that their cavity attenuator acts as a type of Purcell filter:
"the cavity attenuator filters out off-resonance radiation and thus further suppresses the radiative decay of the qubit [11, 12], acting as an effective Purcell filter [39]."
(2) Attenuators have been compared directly to filters and attenuators were better!
In "Paper #2" mentioned above, they did an experiment in which a non-attenuating filter was compared to various attenuators:
"As a control experiment, we also measured an aluminum filter with identical dimensions to the copper attenuator that however
provides no attenuation on resonance."
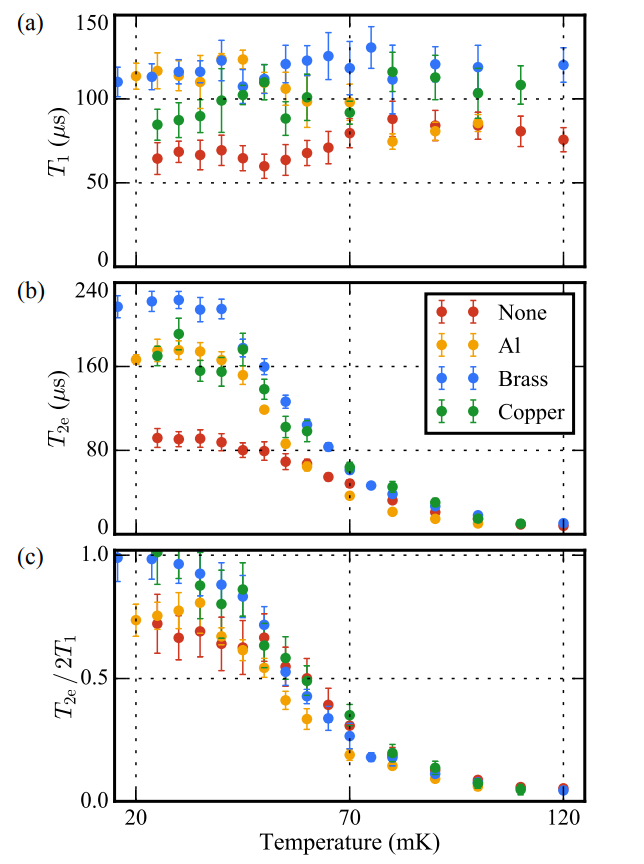
The results show that the non-attenuating (aluminum) filter had significantly faster $T_1$ decoherence times than the brass cavity attenuator beyond about 70 mK, and had up to almost 80 micro-seconds faster $T_2$ decoherence times than the brass cavity attenuator. Furthermore, up to at least 70 mK, the ratio of $T_2$ to $T_1$ was notably worse in the non-attenuating filter compared to both the brass and copper cavity attenuators:
(3) It is not only the frequencies near the qubit frequency which are important
This is for many reasons.
There may be many approximate theories of quantum dissipation, in which the frequencies of the spectral density that are closest to the qubit frequency are the only ones that have a profound effect, but in the formally exact Feynman-Vernon formalism, all frequencies can have an impact on the qubit's decoherence.
If $J(\omega)$ is the spectral density, then the bath response function involving the Bose-Einstein distribution function will be:
$$
\tag{1}
\alpha(t) = \frac{1}{\pi}\int_0^\infty J(\omega)\left( \coth\left( \frac{\beta\omega\hbar}{2} \cos(\omega t) - \textrm{i}\sin(\omega t) \right) \right)\textrm{d}\omega.
$$
Notice that we have integrated over all frequencies. This then enters the Feynman-Vernon influence functional:
$$
\tag{2}
I = \exp\left( -\frac{1}{\hbar}\int_0^t \int_0^{t^\prime} \left( s^+(t^\prime) - s^-(t^\prime) \right)\left( \alpha(t^\prime - t^{\prime \prime})s^+(t^{\prime \prime}) - \alpha^*(t^\prime - t^{\prime \prime})s^-(t^{\prime\prime}) \right) \right).
$$
This single functional, $I$, is 100% of what you need in order to calculate the dynamics of an open quantum system when given the system Hamiltonian, temperature, initial system density matrix, and assuming that $\rho(t=0)$ of the system plus bath is given by:
$$
\tag{3}
\rho(t=0) = \rho_{\textrm{system}}(t=0) \otimes \frac{e^{-\beta H}}{\textrm{tr}\left(e^{-\beta H}\right)},~~~~~~~~\beta\equiv \frac{1}{k_BT}.
$$
This means that the influence functional that tells you how the environment of a qubit affects it, involves a kernel $\alpha(t-t^\prime)$ which does not only depend on the qubit frequency, but involves an integral over all $\omega$.
Another reason is that when you build a quantum computer with 100s of qubits, there's more system frequencies involved than just the single frequency between the two energy levels of a qubit. There's frequencies associated with 2-qubit gates and such, so again the qubit frequency is not the only important frequency.
(4) Dissipation is not always bad.
Most of the papers I've seen talking about attenuation in superconducting qubits are by William Oliver's group at the MIT Lincoln Laboratory, which happens to be a group that is trying to make adiabatic quantum annealers that are like D-Wave's but with also $XX$ coupling (so that they have a better chance at doing universal quantum computation somewhat efficiently). The superconducting qubits at the MIT Lincoln Lab, plus the ones at D-Wave (both of them are aiming to do quantum annealing and not circuit-based quantum computation, constitute a large fraction of all superconducting qubits in the world, and remember that noise can actually help in the annealing process. If you're interested in how dissipation can help with quantum annealing, you might find it more intuitive to first look at:
Even for quantum computing that does not use quantum annealing at all, Paper #2 listed above, says:
"in most of the lines attenuation
and thus dissipation is anyway desired in order to thermalize the incoming
radiation fields, see Section 2.2."
meaning that dissipation is sometimes desired, so you don't want to remove all of it.

