1
$\begingroup$

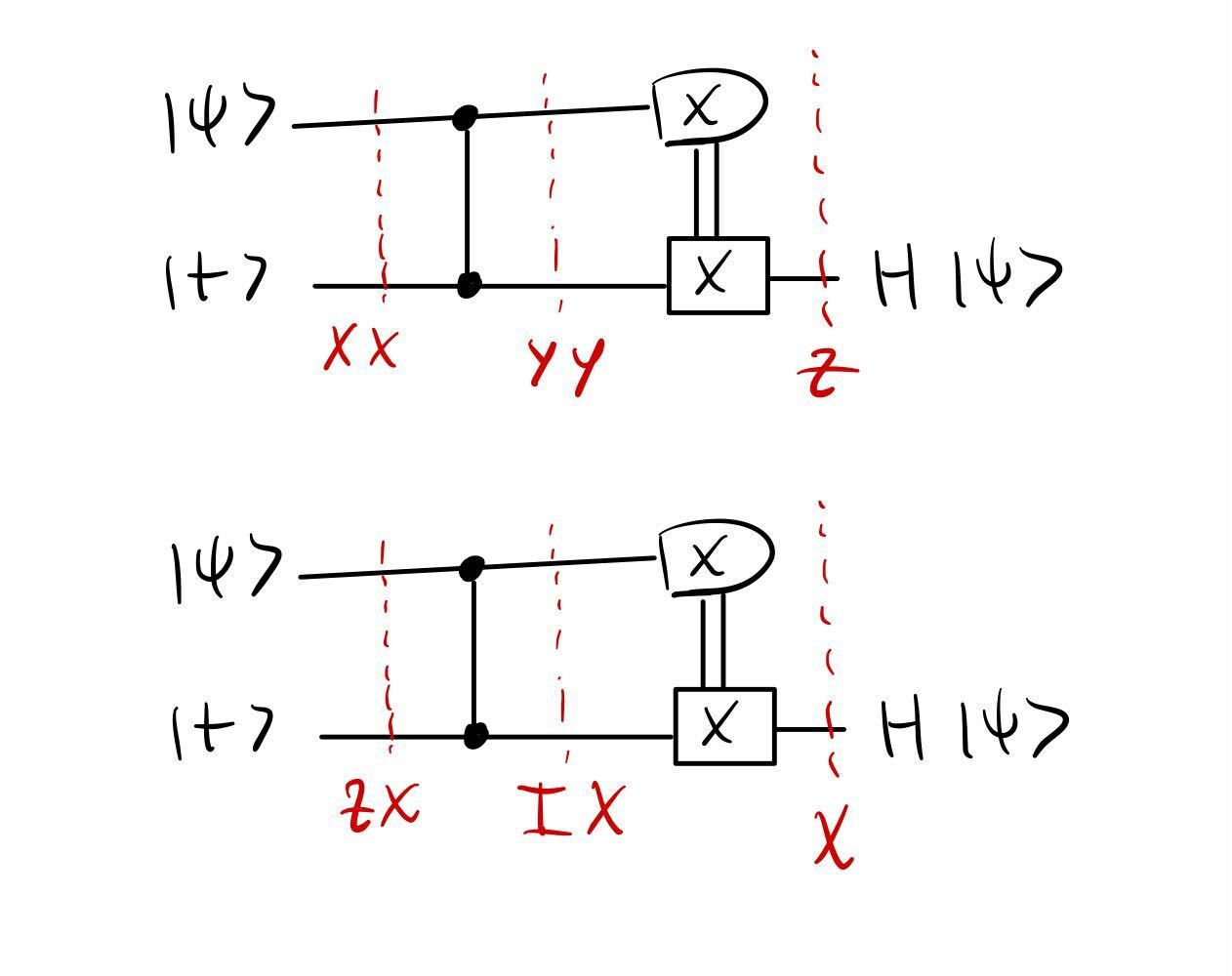
Would somebody please be able to explain the action of this circuit to me? (Taken from Arthur Pesah's blog post "Computing with Quantum Codes using Transversal Gates")

enter image description here

I understand overall that this circuit is implementing the $H$ gate on the qubit $| \psi \rangle$. However, I don't understand how the circuit explicitly implements this logical gate?

I am assuming the two control symbols connecting $| \psi \rangle$ and $| + \rangle$ are entangling the states somehow? Then I don't understand the top right measurement. It is not in a square box, what does this mean? Does it just imply that it is a measurement operator?

I am clearly not well versed in circuit diagrams, and have not had any luck figuring this out myself. If anybody could help break the action of this circuit down for me, it would be very helpful!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

I am assuming the two control symbols connecting |𝜓⟩ and |+⟩ are entangling the states somehow?

Yes, this represents a CZ gate.

Then I don't understand the top right measurement. It is not in a square box, what does this mean? Does it just imply that it is a measurement operator?

Yes, it is a measurement in the X-basis.

You can track $X$ and $Z$ stabilizers on the top qubit to show that the circuit implements a Hadamard gate:

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ This is very helpful! However, I am a bit confused about the action of the circuit on the stabilizers: my understanding from the circuits above is that $CZ(XX) \rightarrow YY$ but that would be the same as multiplying the matrix rep. of $CZ$ ($(1,1,1,-1)$ diagonal matrix ) by the matrix rep of $X \otimes X$ ($(1,1,1,1)$ on the anti-diagonal), which results in $(1,1,1,-1)$ on the anti-diagonal, which does not correspond to $Y \otimes Y$ which is $(-1,1,1,-1)$ on the anti-diagonal. Am I misinterpreting this entirely? $\endgroup$
    – am567
    Commented Feb 16 at 14:19
  • 1
    $\begingroup$ Yes 𝐶𝑍(𝑋𝑋)→𝑌Y is correct, here is a crumble circuit . Tracking stabilizers using the heisenberg representation is not the same as multiplying matrices. This is a good source to learn about the heisenberg representation with some examples: arxiv.org/abs/quant-ph/9807006 $\endgroup$
    – Peter-Jan
    Commented Feb 16 at 14:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.