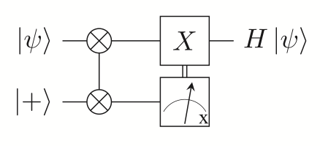
Is the plus state a magic state for the Hadamard gate?

Question

Is the plus state $\left|+\right>:=\frac{\left| 0\right>+\left| 1\right>}{\sqrt{2}}$ a magic state for the Hadamard gate $H$? That is, given the ability to perform (controlled) Pauli operators, can I consume an ancillia prepared with state $\left| + \right>$ to perform the Hadamard gate on another qubit?

To me this seems like a statement which must be true: you are given superposition in the form of $\left| +\right>$, and now all you have to do is transfer that superposition onto another qubit in the simplest way possible. I've been trying to come up with an explicit construction for a while now though, and I can't find one.

At the end of the paper "On the Power of Reusable Magic States", the author asks if there exists a reusable magic state for $H$. This seems to imply that they are aware of the existence of a non-reusable magic state for $H$, which I assume would be $\left| + \right>$.

Answer 1

Start with a state $|\psi\rangle|+\rangle$. Measure the operator $X_1Z_2$ using either lattice surgery or an ancilla qubit and $CZ,CX$ gates. Call this result $m_{xz}$ Then measure the first qubit in the $Z$ basis, call this result $m_z$. The state on the remaining qubit is then

$$ X^{m_{xz}}Z^{m_z}H|\psi\rangle $$ so you have applied an H gate, up to a Pauli correction.

Answer 2

The above circuit shows how to inject a Hadamard gate by means of a magic state $|+\rangle$ and an Ising gate.