6
$\begingroup$

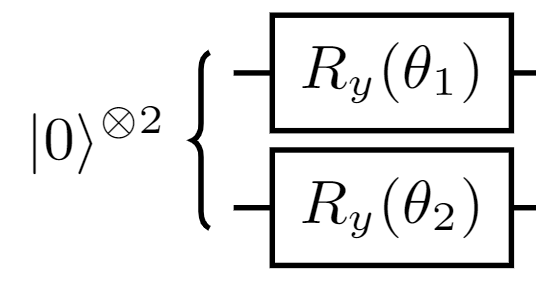
If I have a 2-qubits circuit with a Ry rotation gate acting on each one :

enter image description here

My unitary transformation performed on the 2-qubits state is written as :

$$e^{-i\theta_{1} \sigma_{y}} \otimes e^{-i\theta_{2} \sigma_{y}}$$

I was wondering if I could simplify this product in order to have only one exponential(thus making the tensor product disappear) and what would the result be ?

Improve this question
$\endgroup$
2
  • $\begingroup$ Since the transformation is unitary, this is possible- any unitary can be written as exp(iH) with H an Hermitian operator. $\endgroup$
    – Yaron Jarach
    Commented Apr 19, 2023 at 19:50
  • $\begingroup$ Can you detail how you would do it ? I am still confused about those kind of calculations $\endgroup$
    – Duen
    Commented Apr 19, 2023 at 20:51

1 Answer 1

Reset to default
9
$\begingroup$

The two gates act on separate qubits, so their generators commute and you can use the identity $$ e^A \otimes e^B = e^{A \otimes \mathbb{I} + \mathbb{I} \otimes B} $$ to get $$ e^{-i\theta_1 \sigma_y} \otimes e^{-i\theta_2 \sigma_y} = e^{-i(\theta_1 \sigma_y \otimes \mathbb{I} + \mathbb{I} \otimes \theta_2 \sigma_y)} $$ if that helps simplify things.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thank you for the great answer ! Beginner question : what are the generators of my gates here ? $\endgroup$
    – Duen
    Commented Apr 20, 2023 at 7:14
  • 1
    $\begingroup$ The generator is the operator in the exponent; in your case, the generator of $R_y(\theta)$ is $-\theta \sigma_y$. $\endgroup$
    – Cody Wang
    Commented Apr 20, 2023 at 9:18
  • $\begingroup$ Do I have to also take the "i" or is it useless ? $\endgroup$
    – Duen
    Commented Apr 20, 2023 at 9:20
  • 1
    $\begingroup$ Oops, nice catch; I've added the $i$ to the answer! The $i$ is not considered part of the generator though. $\endgroup$
    – Cody Wang
    Commented Apr 20, 2023 at 9:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.