If you have a general linear system $Mx=b$, then a quantum computer can at best solve the corresponding $$A\left|x\right> = \left|b\right>,$$
where $\left|b\right> = \frac{b}{||b||}$, $A=\frac{M}{||M||}$ is the original matrix normalized so that $||A|| \leq 1$ and $\left|x\right> = \lambda x$.
For VQLS specifically you must further decompose the matrix as a linear sum of unitary matrices $A=\sum_{l} c_l A_l$.
In your case, $$A = c_0 A_0 = 0.5 (X \otimes X \otimes X).$$
As $A_0A_0^{\dagger}=I$ the operations cancel out indeed and the measurement result will be $P(0)=1$.
The circuit you present in your question is only to compute $\left<\psi|\psi\right>$ though, which in your case is 1. As you need to evaluate a normalized cost $$C = \frac{\left< \psi | H | \psi \right>}{\left<\psi|\psi\right>}$$ you have to evaluate the Hamiltonian, global or local, $\left<\psi| H |\psi \right>$, in which case the $A_l$'s never cancel out.
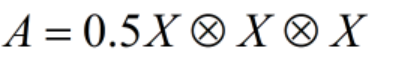
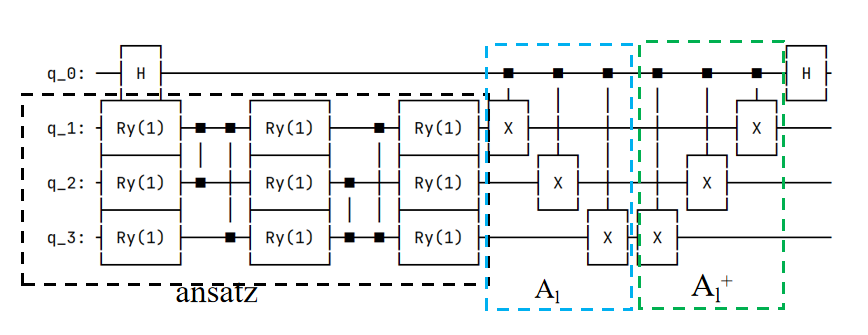 I think this circuit is error, because I can't get the right result... Thanks for any help!
I think this circuit is error, because I can't get the right result... Thanks for any help!